WIXLIB

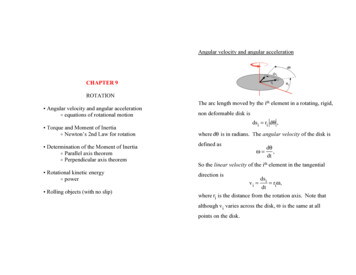
Angular velocity and angular accelerationCHAPTER 9ROTATION Angular velocity and angular acceleration! equations of rotational motion Torque and Moment of Inertia! Newton’s 2nd Law for rotationThe arc length moved by the ith element in a rotating, rigid,non deformable disk isdsi ri dθ ,where dθ is in radians. The angular velocity of the disk is Determination of the Moment of Inertia! Parallel axis theorem! Perpendicular axis theoremdefined as Rotational kinetic energy! powerdirection is Rolling objects (with no slip)ω dθ,dtSo the linear velocity of the ith element in the tangentialvi dsi riω,dtwhere ri is the distance from the rotation axis. Note thatalthough v i varies across the disk, ω is the same at allpoints on the disk.

If the angular velocity changes there is angularAngular velocity ( ω) (vector)acceleration.Dimension: ω 1[T](Check: v i riω [L]1 [L] ).[T] [T]Units: rad/sThe angular acceleration of the disk isα dω d dθ d 2θ ,dt dt dt dt 2and the tangential acceleration of the ith element isdvdωa it i ri riα.dtdtBut, because the ith element is in circular motion, it alsoexperiences a centripetal (radial) acceleration,v2a ic ( a ir ) i riω 2 .riThe resultant linear acceleration is a i a 2it a 2ic .Angular acceleration ( α) (vector)Dimension: α Units: rad/s21[T]2

r 0.12 m: ω! 0: θ! 0: t 5 srα 3.00 rad/s2 .ω ?: θ ?: a t ?: a c ?(a) ω ω! αt (3.00 rad/s2 )(5 s) 15.0 rad/s.(b) v i riω (0.12 m)(15.0 rad/s) 1.80 m/s (linear). tangential acceleration: a t riα (0.12 m)(3.00 rad/s2 ) 0.36 m/s 2 . centripetal acceleration: a c riω 2 (0.12 m)(15.0 rad/s)2 27.0 m/s2 .v 2 (1.80 m/s)2(Check . a c 27.0 m/s2 .)r0.12 m1(c) (θ θ! ) ω! t αt 221 (3.00 rad/s2 )(5 s)2 37.5 rad,237.5 rad n 5.97 rev.2π

As we saw in chapter 4 that force produces change inmotion. However, force does not always produce a[M][L] [M][L]2Dimension: τ [L] [T]2[T]2change in rotational motion. It is torque that produces a(vector)Unit: N mchange in rotational motion. Consider a mass m attachedto a massless rigid rod that rotates around an axis O. Theforce F shown will cause the mass to rotate.Form the radial and tangential components of theforce:"FrO"Fθmℓℓ r sin θrOℓFt F sin θmrO"FθmFr F cos θℓThe magnitude of the torque due to a force F on m is:τ ℓF (r sin θ)F,Then τ ℓF (r sin θ)F r(F sin θ)where ℓ is called the lever arm. The lever arm is theperpendicular distance from the axis of rotation (O) tothe line of action of the force.i.e., τ rFt .Note: the radial component Fr , which passes throughthe axis of rotation, does not produce a torque and"NOTE: if F passes through O, i.e., ℓ 0, then τ 0 andtherefore it does not produce rotation; only thethere will be no change in rotational motion.in rotation.tangential component Ft produces a torque that results

Ft F sin θrO"FθmFr F cos θFrom above, for each separate mass element, we haveτi mi ri2α,ℓNewton’s 2nd Law tells us that the tangential componentof the force Ft produces a tangential acceleration a t,i.e., Ft ma t .Therefore, the torque isτ rFt mra t ,but, from earlier, the tangential (linear) acceleration isrelated to the angular acceleration α, viz: a t rα. τ mra t mr 2α.So torque (τ) produces angular acceleration (α).A rigid object that rotates about a fixed axis can bethought of as a collection of small, individual elementsof mass mi that each move in a circular path of radius ri,where ri is measured from the axis of rotation.riOmiwhere τi is the net torqueon the ith element.Summing over allelements, the total nettorque on the object is:()τ net i τi i mi ri2α i mi ri2 α Iα,where I i mi ri2 is called the MOMENT OF INERTIA.This is Newton’s 2nd Law for rotation, i.e.,τ net Iα.“A net external torque acting on a body producesan angular acceleration, α, of that body givenby Iα, where I is the moment of inertia.”(viz: Fnet i Fi ma .)

Every object has a moment of inertia about an axis. Thevalue depends not only on the mass but how the mass isdistributed around that axis. For discrete collection ofobjects the moment of inertia about the rotation axis isI i I i i m iri2 .For a ‘continuous’ object, where m is a function of r,I Limit i m iri2 r 2dm.m i 0What is the significance of the moment of inertia? MASS a measure of the resistance to a change inlinear motion, e.g., how difficult it is to start, stop orchange linear motion. MOMENT OF INERTIA a measure of theresistance to a change in rotational motion, e.g., howdifficult it is to start, stop or change rotational motion.

Values of the moment of inertia for “simple” shapes .Thin rodA rod is a continuous object, sox ℓI r 2dm 2x ℓx 2dm.Substituting for dm, we obtainbbaa1I Ma 23Thin rod1I ML23Hollow cylinder1I M(R 2 r 2 )Hollow sphere2I MR 223Thin-walled cylinderI MR 2ℓ3 2 x2I x 2 M dx M ℓℓ 3 ℓ 2LSlab( )( ) ( )1I M(a 2 b 2 )12L2The mass per unit length of the rod is M ℓ , so the mass ofthe element dx isdm M dx.ℓℓSlab1I ML2121 Mℓ2 . 12 ℓ2Show for yourselves that the moment of inertia of a rod of1mass M and length ℓ about one end is I Mℓ2 .3Solid cylinder1I MR 22Solid sphere2I MR 25

Ob5 kgaO′The combined moment of inertia is I I ruler I mass ,where I ruler and I mass are calculated about O O′ .Question 9.4: A 1 m ruler has a mass of 0.25 kg. A 5 kgmass is attached to the 100 cm end of the rule. What isits moment of inertia about the 0 cm end?Since a b we assume the meter rule is a rod (viz:1Question 9.2) with I Ma 2 about O O′ .311 I ruler Ma 2 (0.25 kg)(1 m)233 0.083 kg m2 .The moment of inertia of the 5 kg mass about O O′ isI mass ma 2 (5.0 kg)(1 m) 2 5.0 kg m2 .Thus, the total moment of inertia of the ruler and mass isI ruler I mass 5.083 kg m2 .

DISCUSSION PROBLEM [9.2]:DISCUSSION PROBLEM [9.1]:A pair of meter rulers areplaced so that their lowerends are against a wall.One of the rulers has alarge mass attached to itsupper end. If the meterrulers are released at thesame time and allowed tofall, which one hits thefloor first?A: The meter ruler with the mass.B: The meter ruler without the mass.C: They hit the floor at the same time.

Perpendicular axis theorem:zConsider a planar object (e.g., a thinyirimixiydisk or sheet) in the x,y plane. Bydefinition, the moment of inertiaabout the z-axis (perpendicular toxthe plane of the object) isI z i mi ri2 i mi (x i2 y i2 ) i mi x i2 i mi y i2But i mi x i2 I x , i.e., the moment of inertia about x, and i mi y i2 I y , i.e., the moment of inertia about y.Question 9.5: Four masses at the corners of a squarewith side length L 2 m are conncted by massless rods.The masses are m1 m3 3 kg and m2 m4 4 kg.Find (a) the moment of inertia about the z-axis, (b) themoment of inertia about an axis that is perpendicular tothe plane of the ensemble and passes through the centerof mass of the system, (c) the moment of inertia aboutthe x-axis, which passes through m3 and m4.yL I z I x I y .m1Note: the object must be planarm2LExample of a disk:zyx1I z MR 2 .2But, by symmetry, I x I y .1 I x I y MR 2 .4m4m3xz

y2m3 kg2mD2mwith discrete masses4 kgcm4 kgy(a) Since we are dealing2m4 kg2mMoment of inertia about3 kgxz3 kgI i Ii i mi ri2 .xI z i mi ri223 kg4 kgthe z-axis:z2 (3 kg)(2 m) (4 kg)(2 2 m) (3 kg)(2 m)2 (4 kg)(0) 56 kg m2 .(b) By symmetry, the center of mass is at the center ofthe square.(c) Since the ensemble is planar and confined to the x,yplane, we can use the perpendicular axis theorem, i.e.,Iz Ix Iy.But, by symmetry, I x I y .(11 I x I z 56 kg m222 Icm i mi ri2 (3 kg)( 2 m)2 (4 kg)( 2 m)2 (3 kg)( 2 m)2 (4 kg)( 2 m)2 28 kg m2 . Check, using the parallel axis-theoremI z Icm MD2 Icm I z MD 2 (56 kg m2 ) (14 kg)( 2 m)2 28 kg m2 .) 28 kg m2 .Check:I x i mi ri2 (3 kg )(2 m )2 (4 kg )(2 m)2 28 kg m2 .

(a) Using the parallel axiszQuestion 9.6: Four thin rods, each of length ℓ and massM, are arranged to form a square, in the x,y plane, asshown. If the origin of the axes is at the center of thesquare,ℓtheorem, we have for eachℓrodyI z Icm Md 2 ,x(a) using the parallel axis theorem, show that4I z Mℓ 2 .3(b) Hence find I x and I y.zℓ 1ℓ2 4 I z (total) 4 mℓ2 m mℓ2 .4 3 12(b) Since the object is planar we can use the perpendicularℓyxwhere Icm is the moment ofinertia through the center of mass of each rod and d ℓ 2 .axis theorem, i.e.,Iz Ix Iy.But because of symmetry I x I y .12 I x I y I z mℓ2 .23

Rotational kinetic energyIncreasing the rotational kinetic energy of an objectrequires work to be done.An object rotating about an axis has rotational kineticenergy!If the point of application of the force moves a distance d s,then the work done is! !dW F d s Fdscos γ Fcos γds Ft ds Ft rdθ τdθ.Power is the rate at which the torque does work,dWdθi.e., P τ τω.dtdtNote, this is the instantaneous power. dW τdθ is the analog of dW Fds. P τω is the analog of P Fv.

From earlier, power P τω. Convert the torque to N mand angular velocity to rad/s,i.e., τ 1.36 560 762 N m,andω Question 9.7: The 3.9 liter V-8 engine fitted to a 488GTBFerrari develops 560 ft lb of torque at 3000 rev/min.What is the power developed by the engine with theseparameters? (1 ft lb 1.36 N m.)2π(3700 rev/min) 387.5 rad/s.60 s/min P (762 N m)(387.5 rad/s) 2.95 105 watts.But 746 watts 1 HP P 2.95 105 watts 396 HP.746 watts/HP

(a) To find the rotational kinetic energy we need to knowthe angular velocity (ω). Treating the grindstone as aQuestion 9.8: An electric motor exerts a constant torqueof 10.0 N m to the shaft of a grindstone with mass16.0 kg and radius 0.50 m. If the system starts from rest,find(a) the rotational kinetic energy of the grindstoneafter 8.0 s,(b) the work done by the motor during this time, andsolid disk11I MR 2 (16.0 kg)(0.50 m) 2 2.0 kg m2 .22τ 10 N mSince τ Iα, α 5.0 rad/s2 .2I 2 kg mThe grindstone starts from rest ( ω! 0) soω αt (5.0 rad/s2 )(8.0 s) 40 rad/s.11 K Iω2 (2 kg m2 )(40 rad/s)2 1600 J.22(c) the average power delivered by the motor.(b) There are two ways to determine the work done bythe motor.(i) By the work-kinetic energy theorem we wouldexpect the motor to have done 1600 J of work.(ii) We can use the expression W τθ, but we needto find θ, i.e., the angle through which the grindstone hasturned in 8.0 s.11θ ω! t αt 2 (5.0 rad/s2 )(8.0 s)2 160 rad22

11θ ω!t αt 2 (5.0 rad/s2 )(8.0 s)2 160 rad.22pivot W τθ (10.0 N m)(160 rad) 1600 J.(c) The average power is the total work done divided bythe total time interval, i.e.,ΔW 1600 JPav 200 W.Δt8.0 sQuestion 9.9: The “zero” end of a 1 m ruler of massNote: the expression P τω we derived earlier is actually0.25 kg is attached to a frictionless pivot, the other end isthe instantaneous power. We cannot use that expressionfree to rotate in the vertical plane. If the ruler is releasedhere as ω is not constant.from rest in the horizontal position, what isThe instantaneous power actually increases linearly fromzero at t 0 to (10.0 N m) (40 rad/s) 400 W att 8.0 s.(a) the initial acceleration of the 100 cm end of theruler, and(b) the linear speed of the 100 cm end as the rulerpasses through the vertical?

ℓU1 mg : K1 02xcmℓmg(a) Use Newton’s 2nd Law21U2 0 : K 2 Iω 22xfor rotation, i.e., τ Iα,where τ mg ℓ 2 andI 13 mℓ2 . Thenα τ I 3g 2ℓ ,The linear velocity of the 100 cm end as it passes thewhich is the initial angularvertical is then( )acceleration of the center ofmass. The linear acceleration of the 100 cm end is thena ℓα 3g 2 14.7 m/s2 ,which is greater than g! Also note, the torque τ varies asthe ruler swings down.(b) To find the speed of the 100 cm end as it passes thevertical we use the conservation of mechanical energy,i.e., U1 K1 U2 K2.Taking the zero of the gravitational potential energy at thepoint where the center of mass is at its lowest point, thenℓ1mg 0 0 Iω 2 .22 ω mgℓ3g .Iℓv ℓω 3gℓ 3(9.81 m/s 2 )(1.0 m) 5.42 m/s.