WIXLIB

Euler's Pentagonal Number TheoremAuthor(s): George E. AndrewsReviewed work(s):Source: Mathematics Magazine, Vol. 56, No. 5 (Nov., 1983), pp. 279-284Published by: Mathematical Association of AmericaStable URL: http://www.jstor.org/stable/2690367 .Accessed: 20/02/2013 14:16Your use of the JSTOR archive indicates your acceptance of the Terms & Conditions of Use, available at ms.jsp.JSTOR is a not-for-profit service that helps scholars, researchers, and students discover, use, and build upon a wide range ofcontent in a trusted digital archive. We use information technology and tools to increase productivity and facilitate new formsof scholarship. For more information about JSTOR, please contact support@jstor.org.Mathematical Association of America is collaborating with JSTOR to digitize, preserve and extend access toMathematics Magazine.http://www.jstor.orgThis content downloaded on Wed, 20 Feb 2013 14:16:29 PMAll use subject to JSTOR Terms and Conditions

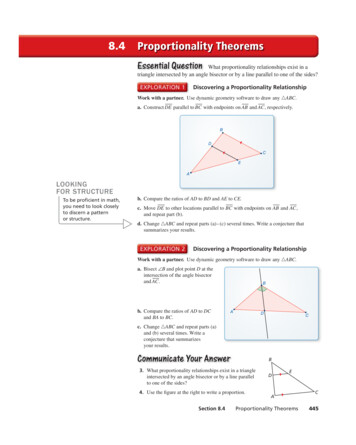
Euler's Pentagonal NumberTheoremGEORGE E. ANDREWSThe PennsylvaniaState UniversityPark, PA 16802UniversityOne of Euler's most profounddiscoveries,the PentagonalNumberTheorem[7], has beendescribedby AndreWeil:beautifullyPlayingwith series and products,he discovereda numberof factswhich to him lookedHe looked at thisinfiniteproductquite isolatedand verysurprising.(I--x)(X)(-X3).startedexpandingit. He had manyproductsand seriesof thatkind; in someand just formallycases he got somethingwhichshowed a definitelaw, and in othercases thingsseemed to beratherrandom.But with this one, he was verysuccessful.He calculated at least fifteenortwentyterms;the formulabeginslike this:1 -x-X2 1I(1 -Xn) X5 x7-x12X15where the law, to your untrainedeyes, may not be immediatelyapparentat firstsight.Inmodem notation,it is as follows: 0000I(I-q1n) E:(I)n qn(3n 1)/2(1-00whereI've changedx intoq sinceq has becomethestandardnotationin ellipticfunction-theorysince Jacobi. The exponentsmake up a progressionof a simple nature. This became immediatelyapparentto Euler afterwritingdown some 20 terms;quite possiblyhe calculatedabout a hundred.He veryreasonablysays,"this is quite certain,althoughI cannotproveit;"ten yearslaterhe does proveit. He could not possiblyguess thatboth seriesand productareandIt is anothertie-upbetweennumber-theorypartof thetheoryof ellipticmodularfunctions.ellipticfunctions[22,pp. 97-98].discoveryG. P6lya [16, pp. 91-98] providesa moreextensiveaccountof Euler'swonderfulof Euler'sowndescriptionwitha translationtogether[6].toThe numbersn(3n - 1)/2 are called "pentagonalnumbers"because of theirrelationshipthis.Legendre[14,pp. 131-133]observedthatpentagonalarraysof points.FIGURE 1 illustratesof thetermson theleftside of (1) producestheterm qN preciselypurelyformalmultiplicationthe" " is takenwhenthereispositiveintegers;as a sumofdistinctas oftenas N is representable"-"thewhenthenumberof summandsinandtherepresentationan evennumberof summandsis odd. For example,n 1n 3n 2n 4FIGURE 1. The firstfourpentagonalnumbersare 1, 5, 12, 22.VOL. 56, NO. 5, NOVEMVBER1983This content downloaded on Wed, 20 Feb 2013 14:16:29 PMAll use subject to JSTOR Terms and Conditions279

(1 -q)(I- q3)(1-q2)(1Ilqq2q31q 2 3 q3 4 q2 4.- q4)q4 q1 2 q1 3 q1 4 q2 31q1 2 41q 3 42 3 41 q1 2 3 4 ofa positiveintegeras thesumofis usuallyusedto describetherepresentationThe termpartitionIn thisarticle,we are positiveintegers.thesameifthetermsin thesumare thesame,e.g.,1 2 and 2 1 are consideredare consideredas the same partitionof 3. Thus Legendre'sobservationmay be matchedup withthe actualinfiniteseriesexpansion(1) as follows.of n intoan even numberof distinctTHEOREM.Let pe(n) denotethe numberof partitionsofdistinctsummands.Letpo(n) denotethenumberofn intoan oddnumberofpartitionssummands.Thenp,e(n)n-p(n) -po(n (2)ifn j(3j?1)12((-Wotherwise.on subseThe impactof Euler'sPentagonalNumberTheoremand Legendre'sobservationsBoth(1) and (2) arejustlyfamous.Indeed,F.in numbertheoryis enormous.quentdevelopmentsproofof(2) [10](see also [21,pp. 261-263])has n'sproofis soof Americanmathematics.achievementRademacheras thefirstsignificantalgebraandand lovelythatit has been presentedmanytimesoverin elementaryelementarynumbertheorytexts[5,pp. 563-564],[11],[12,pp. 206-207],[13,pp. 286-287],[15,pp. 221-222].It is, however,to notethatEuler'sproofof (1) alludedto by Weil remainsalmostinterestingunknown.In recentyearsonlyRademacher'sbook has containedan expositionof it [17, pp.it moreor less as Eulerdid.224-226].Thisbook and earlierbooks[4,pp. 23-24] havepresentednotationWhiletheidea behindEuler'sproofis ingenious(as one wouldexpect),themathematicalofcorollariesare eithertransparentof Euler'sday hidesthefactthatotherresultsof significanceof thisarticleis devotedto a longEuler'sproofor lie just below the surface.The remainderof itsconsequences.overduemodemexpositionof Euler'sproofand an examinationof twovariables:To begin,we definea function00E, q)f(n Ixq)(I(Il- xq.2)I**(-xqt-)X n 1qn.3is ensuredby Iq I 1, Ix jof all seriesand t00f(I, q) Hn I(1)qlqWe(4)- qt").Thisis becausewe mayeasilyestablishtheidentityI-NEn I(I- q)(IN- q2).(I - qt-1) qtlHII I(I - qn)(5case of(5)on N (a niceexerciseforthereader).Thus(4) is thelimitinginductionby mathematicalas N- oo.of the followingfunctionalThe main step in Euler's proofis essentiallythe verificationequation:f (x q) 1 - x2q - x3q2f(xq q).(6)MATHEMATICS MAGAZINE280This content downloaded on Wed, 20 Feb 2013 14:16:29 PMAll use subject to JSTOR Terms and Conditions

ActuallyEulerdoes equation(6) overand over,firstwithx 1,thenx q, thenx q2, and so on[7,pp. 473-475];page473 ofhispaperin OperaOmniais shownon thenextpage.ThisrepetitionTo prove(6), we takethedefiningof specialcases of (6) tendsto hidewhatexactlyis happening.a sequenceof algebraicmanipulations:equation(3) through00f(xgqn 2.-Xq)(I1 -xq 2)(IEI X2q-) Xn l q nn-(I -xq00 -x2q- x 2q-E (1*- (I-xq2)-xq)(In 1-xqn)Xn 2qn 00En 1oo2 q- -E(I - xq2)(I -xq2.(I-xq n) xn 2q) .(I-xqn)-- xq)II I(IX n 22q n 1n 1oo En 1(1 -xq2)-xqn)xn 3qn 2(I*.00 3q 2-2q-x-x,-(In 2xq2).(I - xqn 2)xqI Ioo E.(I -xq2)n Ixq")Xn 3qn 2(I00I -x2q-x3q2- En 1n 1.(1 -xq2))X* (I -xqn(I -xq 2)-qxqn)xn 3qn 2(I00Ix 2q-x3q2En 13q 2 { Ix 2q-x 1-x2q-x3q2f(I -xq 2)-E (In 1.(I-xq 2) .(1-xqn)Xn 3xqqn 2((ln)x,,1xq n l1)q2n)?I(xq, q)-yousee howat each stagethingsseemtoIf callyat just the rightmoment.Also you can appreciatethe complicationfittogetherofthesamestepsfirstwithx 1,thenx q, thenx q2, etc.However,therepeatedpresentationmanner.in thisrepetitiveof (6) by Eulermusthave comepreciselyempiricaldiscoveryiterated;thusThe restof Euler'sproofis now almostmechanical.Equation(6) is repeatedlyf (x,1X2q-X3q2(1q) l1-x2q-x3q2X2q3 X3q5f(xq2q)) x5q5 x6q7(l X2q5 x3q8f(xq3, q))(7)N-i 1 n(3n 1)/2)( I)n(X3n-lqn(3n-1)/2 X31qn 1 (1)Nx3N-lqN(3N-1)/2 (-)Nx3NqN(3N VOL. 56, NO. 5, NOVEMBER 1983This content downloaded on Wed, 20 Feb 2013 14:16:29 PMAll use subject to JSTOR Terms and Conditions1)/2f ( xqN, q)281

EVOLUTIO PRODUCTI INFINITI (I -x)(l47-48]-xx)(I-x')(l-zx)473etc.2. Quoniam hi termini omnes factoremhabent communem1 - x, eoevoluto singuli terminidiscerpenturin binas partes, quas ita repraesentemusA - xx x(l - xx) x'(1 - xx)(1 - x) x'(1 - xx)(1 - x)(1 - x') etc.-x-.x' (1-zx)-x5(1-xx)(1-x)-x (1-xx)(1-x')(1-x')-etc.Hinc iam binae partes eadem potestateipsius x affectaein unam contrahantur ac resultabitpro A sequens formaA -xx- x6-x(1-xx) -x(1-xx )- x"(1 - x)(1 - x(l(1-x)-') -etc.,ubi duo terminiprimi xx - x5 iam sunt evoluti; sequentes autem proceduntper has potestatesx7, x9, x11,x S, x15, quarum exponentesbinario crescunt.3. Ponamus nune simili modo ut anteA{ xxita ut sitB x7(1-xx) x1-x5- B,1 - x) (1-x3) xl(l -xx) (t -x)(1 - Z') etc.,cuius omnes terminihabent factoremcommunem1 - xx, quo evolutosinguliterminiin binas partes discerpantur,uti sequitur,B 7 x9(1 - x8) xll(l - x3)(1-- x') x13(1-x3X (--x)3( - x) -ll(l-1l(1(1--xl3)(1-x4)(1 - x) etc.4)(1 - x5) - etc.Hic iterumbini termini,qui eandem.potestatemipsius x habent praefixam,in unam colliganturet prodibitB x7- x1-x5(1 - x) - x18(1- x3)(1 - x4)-x2(1-x)(1-z4)(1-x)-etc.ubi iani potestatesipsius x crescuntternario.4. Statuaturnunc porroB x7- xs - C,ita ut sitC-2']5(x5) v,18(1Xt)(1 ---X ( X-Ix) (1-I5) S),4) j21( etc.,60arithucticaeEut.umOperaoinniaI3 (CommentationesLRONnqitminductionon N. In thelimitas N - oo we findanotherfineexercisein mathematical00f(x, q) 1 En 1(-1)n(X3n- lqn(3n-1)/2 X3nqn(3t 1)/2)(8)Thereforeby (4) and (8),0000Hn l(I- qt') f (I, q) ,: (n lI)n(qn(3n -1)/2 qnl(3n 1)/2)00( n -ool)qn(3n 1)/2,whichcompletestheproofof (1).[19],andIt shouldbe notedthatseveralauthors(L. J.Rogers[18,pp. 334-335],G. W. Starchernonehas notedthatin thewaywe have;however,(8) essentiallyN. J.Fine[9]) also foundformulaEuler'sproofin simplerclothing.M. V. Subbarao[20] has alsohe was, in fact,rediscoveringbetween(8) and THEMATICS MAGAZINE282This content downloaded on Wed, 20 Feb 2013 14:16:29 PMAll use subject to JSTOR Terms and Conditions

ask: Was anythinggainedin thisgeneralformulationof Euler'sNow thereadermaynaturallyIs anynewinformationofpresentation?availablethatwas hiddenbefore?proofbesidessimplicitygivesthex -1 in (3) and (8). ThissubstitutionYES justby settingWe can answera resoundingequation001 (1 q)(1 q2)E(1 qn1)(-n 1 f -1,q) 1 En I(q n(3n-1)/2 l)nqn(9)qn(3n 1)/2)as appealingas Legendre'sTheorem.It impliesthattheproductEquation(9) yieldsa corollary(I q)(I q 2) .(I q n- I)qnintodistinctoutproducesthetermqN exactlyas oftenas N can be partitionedwhenmultipliedpartequalto n. For example,whenn 4,withlargestsummands(1 q)(I q2)(1 q3)q4 q4 q4 1 q4 2 q43 q4 2 1 4 3 1 q4 3 2 q4 3 2 ?1Hence theserieson theleftsideof (9) whenexpandedout yieldstheterm? qN foreachpartitionsummands;the" " occursifthelargestsummandis even,and the" - " occursofN intodistinctif thelargestsummandis odd. In thesamemannerthat(1) yieldedLegendre'sTheorem,we seeequallyelegantbut littlepublicizedresultfoundby N. J. Fine [8]that(9) bservation.thelargestofsummandsofn withdistinctofpartitionsTHEOREM. Let 7r,(n)denotethenumbersummandsthelargestofofn withdistinctofpartitionswhichis even.Let 7r0(n)denotethenumberwhichis odd. Thenqre(n)-gT (n) {1-1Oifn j(3j 1)/2,ifn j(3j- 1)/2,otherwise.partsare:ofn 12 intodistinctwithan example.The partitionsLet us checkoutthetheorems7 3 2, 6 5 1,8 4, 8 3 1, 7 5, 7 4 1,12, 11 1, 10 2, 9 3, 9 2 1,enumeratedby each ofp,(l2),6 4 2, 6 3 2 1, 5 4 3, 5 4 2 1. The partitionsinthetable:areandp0(12), g7,(12)g7r(12) listedfollowingP,1 2)(po(12)11 110 29 38 47 56 3 2 15 4 2 1129 2 18 3 17 4 17 3 26 5 16 4 25 4 3Thuspe(12)-p0(12) 7 - 8 rems.qre( 12)1210 28 48 3 16 5 16 4 26 3 2 1- 1 and 7r,(12)- 7r.(12) 7 - 8--qro( 12)11 19 39 2 17 57 4 17 3 25 4 35 4 2 1.1, as predicted by our pleasantdiscoveryBeyondthisimmediateextensionsof Euler'smethodthatyieldmoreFine's Theorem,we mayask: Are thereinterestingunawarethanequation(8)? Hereagaintheansweris positive.L. J.Rogers[18,p. 334],apparentlyVOL. 56, NO. 5, NOVEMBER1983This content downloaded on Wed, 20 Feb 2013 14:16:29 PMAll use subject to JSTOR Terms and Conditions283