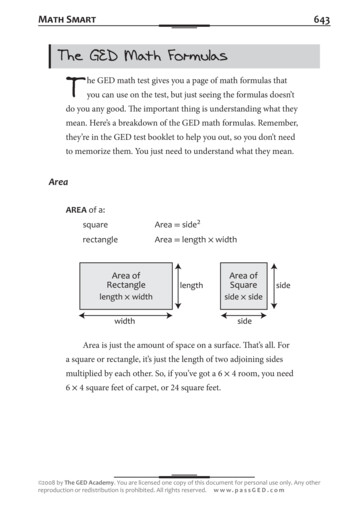
Transcription
PT11/14/0611:23 AMPage 131Find an equation for the line passing throughH(8, 3) with slope 29 .y 32 2 99x 89(y 3) 2(x 8)the isosceles right triangle, and the diagonal is thehypotenuse.9y 27 2x 16243y 9 x 9 IMPROVING YOUR REASONING SKILLSBeginner Puzzle: green left, red up, red right, reddownIntermediate Puzzle: orange down, orange right,red up, red right, red downAdvanced Puzzle: orange down, orange left, red up,red right, yellow down, yellow left, red down9. Always true by the Parallelogram Opposite AnglesConjecture10. Sometimes true. It is true only if the parallelogramis a rectangle. Consecutive angles of a parallelogramare always supplementary, but are only congruent ifthey are right angles.truefalseLESSON 5.6EXERCISES1. Sometimes true. It is only true if the parallelogramis a rectangle.truefalse2. Always true. By the definition of rectangle, all theangles are congruent. By the Quadrilateral SumConjecture and division, each angle measures 90 ,so any two angles of a rectangle, including consecutive angles, are supplementary.3. Always true by the Rectangle Diagonals Conjecture4. Sometimes true; it is only true if the rectangle is asquare.truefalse11. 20. By the Rectangle Diagonals Conjecture, the diagonals of a rectangle bisect each other and arecongruent. By the “bisect each other” part, KC CR 10, so KR 20, and by the “are congruent”part, WE KR, so WE 20.SOLUTIONS MANUALDG4CS 898 C.qxd12. 37 . By the Parallelogram Consecutive AnglesConjecture, P and PAR are supplementary, so48 (y 95 ) 180 . Then y 95 132 ,so y 37 .13. x 45 , y 90 . A diagonal divides a square intotwo isosceles right triangles, so x 45 . Thediagonals of a square are perpendicular (SquareDiagonals Conjecture), so y 90 .14. DIAM is not a rhombus because it is not equilateraland opposite sides are not parallel. You can useslopes to determine that opposite sides of thisquadrilateral are not parallel, so it is not evena parallelogram.15. BOXY is a rectangle because its adjacent sides areperpendicular.5. Always true by the Square Diagonals Conjecture6. Sometimes true. It is true only if the rhombus isequiangular.truefalse7. Always true. All squares fit the definition of rectangle.8. Always true. All sides of a square are congruent andform right angles, so the sides become the legs ofDiscovering Geometry Solutions Manual16. Yes. TILE is a rhombus, and every rhombus isa parallelogram.17. Possible construction: You knowEVby the Square DiagonalsConjecture that the diagonalsof a square are perpendicular .and congruent. Duplicate LVLOConstruct its perpendicularbisector. From the intersectionpoint of the diagonals, set the radius of yourcompass at 12 (LV ). Draw a circle with this centerand radius. Label the two points where the circle as Eintersects the perpendicular bisector of LVand O. Connect the points L, O, V, and E to formthe square.CHAPTER 597 2008 Key Curriculum PressDISCOVERING GEOMETRY COURSE SAMPLER131
DG4CS 898 C.qxd11/14/0611:23 AMPage 13218. One possible construction: Duplicate B. Bisect it.Mark off distance BK along the bisector. At K dupli .cate angles of measure 12 m B on either side of BKLabel as A and E the intersections of the rays of B .with the rays of the new angles which are not BKA21. If the diagonals are congruent and bisect each other,then the room is rectangular (Rectangle DiagonalsConjecture).KBEAnother possible construction: Duplicate B andthen bisect it. Mark off the length BK on the anglebisector. Then construct the perpendicular bisector . Label the points where this perpendicularof BKintersects the sides of B as E and K. Connect thepoints B, A, K, and E to form the rhombus.AKBESOLUTIONS MANUALE19. Possible construction: DuplicateI and construct a perpendiPS through S. Opencular to PSyour compass to radius PEand draw an arc intersectingPSthe perpendicular line thatyou have constructed. Label the intersection pointof the arc and the perpendicular line as E.Construct arcs of length PI and EI. Label theirintersection as I. Connect the points P, I, E, and Sto form the rectangle.20. Converse: If the diagonals of a quadrilateral arecongruent and bisect each other, then the quadrilateral is a rectangle.Given: Quadrilateral ABCD with diagonals BD . AC and BD bisect each otherACShow: ABCD is a rectangleA87D12E6522. The platform stays parallel to the floor becauseopposite sides of a rectangle are parallel. (The Parallelogram Opposite Sides Conjecture applies becausea rectangle is a parallelogram.)23. The crosswalks form a parallelogram: The streets areof different widths, so the crosswalks are of differentlengths. The streets would have to cross at rightangles for the crosswalks to form a rectangle. Thestreets would have to cross at right angles and also beof the same width for the crosswalk to form a square.24. Place one side of the ruler along one side of theangle. Draw a line with the other side of the ruler.Repeat with the other side of the angle. Draw a linefrom the vertex of the angle to the point where thetwo lines meet.25. Rotate your ruler so that each endpoint of thesegment barely shows on each side of the ruler.Draw the parallel lines on each side of your ruler.Now rotate your ruler the other way and repeat theprocess to get a rhombus. The original segment isone diagonal of the rhombus. The other diagonalwill be the perpendicular bisector of the originalsegment.B34CParagraph Proof: Because the diagonals are BE congruent and bisect each other, AE EC . Using the Vertical Angles Conjecture,DE AEB CED and BEC DEA. So AEB CED and AED CEB by SAS. Using theIsosceles Triangle Conjecture and CPCTC, 1 2 5 6, and 3 4 7 8. Eachangle of the quadrilateral is the sum of two angles,one from each set, so for example, m DAB m 1 m 8. By the addition property of equality,m 1 m 8 m 2 m 3 m 5 m 4 m 6 m 7. So m DAB m ABC m BCD 98m CDA. So the quadrilateral is equiangular. Using CD . Using 1 5 and the Converse of AIA, AB AD . 3 7 and the Converse of AIA, BCTherefore ABCD is an equiangular parallelogram, soit is a rectangle.CHAPTER 526. 2. Given; 4. SSS; 5. CPCTC; 9. Definition of rhombus27. Yes, it is true for rectangles.Given: 1 2 3 4Show: ABCD is a rectangleParagraph Proof: By the Quadrilateral SumConjecture, m 1 m 2 m 3 m 4 360 .Because all four angles are congruent, each anglemeasures 90 . Because 4 and 5 form a linearDiscovering Geometry Solutions Manual 2008 Key Curriculum Press132DISCOVERING GEOMETRY COURSE SAMPLER
11/14/0611:23 AMPage 133pair, m 4 m 5 180 . Substitute 90 for m 4and solve to get m 5 90 . By definition ofcongruent angles, 5 3, and they are alternate BC by the Converse of theinterior angles, so ADParallel Lines Conjecture. Similarly, 1 and 5 are CD by thecongruent corresponding angles, so ABConverse of the Parallel Lines Conjecture. Thus,ABCD is a parallelogram by the definition of parallelogram. Because it is an equiangular parallelogram, ABCD is a rectangle.28. a 54 , b 36 , c 72 , d 108 , e 36 ,f 144 , g 18 , h 48 , j 48 , and k 84 .First, a 54 (CA Conjecture). Now look at theright triangle in which the measure of one of theacute angles is b. The other acute angle in thistriangle is the vertical angle of the angle of measurea, so its measure is 54 , and b 90 54 36 .Next, 2c b 180 , so 2c 144 , and c 72 .The angle with measure d forms a linear pair withthe alternate interior angle of the angle withmeasure c, so d 180 72 108 . In thetriangle that contains the angle with measure e,both of the other angle measures have been foundto be 72 , so this is an isosceles triangle with vertexangle of measure e. Therefore, e 2 72 180 ,so e 36 . (Or, by the CA Conjecture, e b 36 .)Now look at the quadrilateral that contains theangle with measure f. This is a parallelogrambecause both pairs of opposite sides are parallel(from the given pairs of parallel lines). Notice thatthe angle that forms a linear pair with the angle ofmeasure e is the angle opposite the angle ofmeasure f in the parallelogram. Therefore, by theParallelogram Opposite Angles Conjecture and theLinear Pair Conjecture, f 180 e 144 . Nowlook at the isosceles triangle in which g is themeasure of a base angle. In this triangle, the vertexangle measures 144 (Vertical Angles Conjecture), so2g 144 180 ; then 2g 36 , and g 18 .Along the upper horizontal line, there are threemarked angles, all of measure h, and one unmarkedangle. The unmarked angle is the alternate interiorangle of the supplement of the angle of measure f,so its measure is 180 144 36 . Then 3h 36 180 , so 3h 144 , and h 48 . Next, j isan alternate interior angle of one of the angles ofmeasure h, so j 48 . Finally, k j 48 180 (Triangle Sum Conjecture), so k 84 .29. Possible answers: (1, 0); (0, 1); ( 1, 2); ( 2, 3).Find an equation for the line passing through thepoints (2, 1) and ( 3, 4), and then find points on4 ( 1)5 that line. First find the slope: m 5 3 2 1. Now use the slope and one of the points on theline to find an equation for the line. Here, (2, 1)is used.Discovering Geometry Solutions Manualy ( 1) x 2 1y 1 x 2 1y 1 1(x 2)y 1 x 2y x 1To find points on this line in addition to the twothat are given, substitute any number for x otherthan 2 and 3 into the equation y x 1 tofind the corresponding value for y. If x 1, y 0;if x 0, y 1; if x 1, y 2; if x 2,y 3. Therefore, four additional points on the lineare (1, 0), (0, 1), ( 1, 2), and ( 2, 3). Any three ofthese points would be sufficient; there are infinitelymany other points on the line.30. y 89 x 89 6 , or 8x 9y 86. The perpendicularbisector of the segment with endpoints ( 12, 15) and(4, 3) is the line through the midpoint of this segmentand perpendicular to it. First find the midpoint: 12 4 15 ( 3) 8 12 , , ( 4, 6) 222 2SOLUTIONS MANUALDG4CS 898 C.qxdThe slope of this segment is 189 3 15 1684 ( 12)So, its perpendicular bisector will have slope 98 . Findan equation of the line with m 98 through ( 4, 6).y 68 x ( 4) 9y 6 8 x 4 99(y 6) 8(x 4)9y 54 8x 32886y 9 x 9 , or 8x 9y 8631. y 17 0 x 15 2 , or 7x 10y 24. The median to goes through C(8, 8) and the midpoint of AB ,ABwhich is ( 2, 1). Find an equation of the line thatcontains (8, 8) and ( 2, 1). First find the slope. 8 ( 1)7 m 8 ( 2) 10Now use the slope and either point to find the equation of the line. Here, the point ( 2, 1) is used.y ( 1) 7 x ( 2)10y 1 7 x 21010(y 1) 7(x 2)10y 10 7x 14712 7x 10y 24, or y 1 x0 5CHAPTER 599 2008 Key Curriculum PressDISCOVERING GEOMETRY COURSE SAMPLER133
DG4CS 898 C.qxd11/14/0611:23 AMPage 13432. Velocity 1.8 mi/h. Angle of path 106.1 clockwise from the north.Because both pairs of opposite sides are parallel,WATR is a parallelogram.2 mi/h60 4. Flowchart Proof1 SP OA2 SP OAGivenGiven1.5 mi/h3IMPROVING YOUR VISUAL THINKING SKILLS5 SOP APOSAS 1 26 3 4AIA Conjecture4 OP OPThe matching pairs are (A1, B3), (B1, D3), (A2, C2),(B2, C4), (A3, B4), (C1, C3), (A4, D2), and (D1, D4).CPCTC7 PA SOSame segmentLESSON 5.7Converse ofAIA Conjecture8Definition of parallelogramEXERCISESSOLUTIONS MANUAL SK ,1. Completion of flowchart proof: 3. OA SA ,definition of parallelogram; 5. AIA; 6. SASame segment; 7. SOA AKS by ASAParagraph Proof: By the definition of a KA and OA SK . Because 3parallelogram, SOand 4 are alternate interior angles formed when and KA are cut by thethe parallel lines SO , 3 4 by the AIA Conjecture.transversal SASimilarly, 1 and 2 are alternate interior angles and SK are cut byformed when parallel lines OAthe same transversal, so 1 2 by the AIA is a shared side of the two triangles;Conjecture. SAevery segment is congruent to itself. Therefore, SOA AKS by ASA.2. Completion of flowchart proof: 4. Given; 5. THA,Conjecture proved in Exercise 1; 6. HBA ATHby CPCTC dividesParagraph Proof: Diagonal BTparallelogram BATH into two congruent trianglesbecause either diagonal of a parallelogram dividesthe parallelogram into two congruent triangles.(This is the conjecture that you proved inExercise 1.) Therefore, BAT THB, and divides BAT THB by CPCTC. Also, HTparallelogram BATH into two congruent trianglesfor the same reason, so BAH THA, and HBA ATH by CPCTC. RT ,3. Completion of flowchart proof: 1. WA AT , Given; 3. WT WT , SameGiven; 2. WRsegment; 4. WRT TAW by SSS; 5. 1 2 WA by Converse of theby CPCTC; 6. RTParallel Lines Conjecture; 7. 4 3 by CPCTC; TA by Converse of the Parallel Lines8. RWConjecture; 9. Definition of parallelogram RT andParagraph Proof: Because WA AT (both given) and also WT WT ,WR WRT TAW by SSS. Then 1 2 by WA by the Converse of the ParallelCPCTC, so RT TA .Lines Conjecture. Similarly, 3 4, so RW100CHAPTER 5SOAP is a parallelogram5. parallelogram6. Flowchart Proof12 GO IYOpposite sides ofrectangle are congruent.3 YO YO GOY IYODefinition of rectangle4Same segment GOY IYOSAS5 IO YGCPCTCParagraph Proof: Look at two overlapping GO becausetriangles: GOY and IYO. IYopposite sides of a rectangle are congruent.(Opposite sides of a parallelogram are congruent,and a rectangle is a parallelogram.) GOY IYOby the definition of a rectangle. (All angles of arectangle are congruent.) Therefore, GOY OI by CPCTC. IYO by SAS, and YG7. Flowchart Proof1 BEAR is a parallelogram.Given2 AB RE3 BR EA Given4 AR EB Opposite sides ofparallelogram arecongruent.5Opposite sides ofparallelogram arecongruent. EBR ARB RAE BEASSS6 EBR ARB RAE BEACPCTC7BEAR is a rectangleDefinition of rectangle AB is given, andParagraph Proof: RE EA and AR EB because opposite sides ofBRa parallelogram are congruent (ParallelogramOpposite Sides Conjecture). Therefore,Discovering Geometry Solutions Manual 2008 Key Curriculum Press134DISCOVERING GEOMETRY COURSE SAMPLER
11/14/0611:23 AMPage 135Quadrilateral Sum ConjectureWrite a proof of the Quadrilateral Sum Conjecture.Conjecture: The sum of the measures of the four interiorangles of any quadrilateral is 360 .Dd ea AQ qTEACHING AND WORKSHEET MASTERSDG4CS 898 C.qxduvUGiven:Quadrilateral QUAD with an auxiliary line, , and angles labeled as showndiagonal DUShow:m Q m U m A m D 360 .Proof76LESSON 5.1 WorksheetDiscovering Geometry Teaching and Worksheet Masters 2008 Key Curriculum PressDISCOVERING GEOMETRY COURSE SAMPLER135
DG4CS 898 C.qxd11/14/0611:23 AMPage 136h 77 122 116 98 1 2edp36 a138 b116 fgc106 TEACHING AND WORKSHEET MASTERSn m60 kj87 2 1Exercise 12Discovering Geometry Teaching and Worksheet Masters 2008 Key Curriculum Press136DISCOVERING GEOMETRY COURSE SAMPLERLESSON 5.1 Transparency77
Discovering Geometry Teaching and Worksheet Masters321LESSON 5.3 TransparencySame segment GivenEN YNGivenBE BY4 2 5 1 andETEACHING AND WORKSHEET MASTERS Congruenceshortcut BEN 216Definition ofangle bisector and 43N11:23 AMFlowchart ProofBY11/14/06 bisects BShow: BN bisects NBN BY Given: Kite BENY with BE YN and ENDG4CS 898 C.qxdPage 137Exercise 9 2008 Key Curriculum Press79DISCOVERING GEOMETRY COURSE SAMPLER137
DG4CS 898 C.qxd11/14/0611:23 AMPage 138៝Vc៝VbExercises 9 and 109.៝Vh៝Vw10.TEACHING AND WORKSHEET MASTERSDiscovering Geometry Teaching and Worksheet Masters 2008 Key Curriculum Press138DISCOVERING GEOMETRY COURSE SAMPLERLESSON 5.5 Transparency83
11/14/0611:23 AMPage 139Chapter 5 Quiz 1Form ANamePeriodDateComplete each statement.1. The sum of the measures of the n interior angles of an n-gonis .2. The number of triangles formed in a decagon when all the diagonalsfrom one vertex are drawn is .3. The sum of the measures of the exterior angles of a 25-gonis .4. The measure of one angle in a regular octagon is .5. If the measure of one exterior angle of a regular polygon is 24 , thenthe polygon has sides.Find each lettered angle measure.ASSESSMENT RESOURCESDG4CS 898 C.qxd6. a b cc 7. m mn rp r bad dtnsps t 72 85 Discovering Geometry Assessment ResourcesLESSONS 5.1, 5.265 2008 Key Curriculum PressDISCOVERING GEOMETRY COURSE SAMPLER139
DG4CS 898 C.qxd11/14/0611:23 AMPage 140Chapter 5 Quiz 2Form BNamePeriodDateFor Problems 1–6, find the lettered measures in each figure. The figures inProblems 1 and 2 are kites.1. w x 2. w x y z x51 4 cmxyy zww72 9 cmy75 148 The figures in Problems 3 and 4 are isosceles trapezoids.ASSESSMENT RESOURCES3. x 4. Perimeter 111 cmy x 23 cmxy119 x30 cm5. x 6. The figure is a trapezoid.y q z q1617xy23132260 40 z68LESSONS 5.3, 5.4Discovering Geometry Assessment Resources 2008 Key Curriculum Press140DISCOVERING GEOMETRY COURSE SAMPLER
11/14/0611:23 AMPage 141Chapter 5 TestForm ANamePeriodDatePart AComplete each statement. Do not use square as an answer.1. In an isosceles trapezoid, the base angles are .2. Each interior angle of a regular decagon measures .3. The vertex angles of a kite are by the diagonal.4. The consecutive angles of a parallelogram are .5. The length of a midsegment between two sides of a triangleis the length of the third side.6. The sum of the measures of the interior angles of a hexagonis .7. The nonvertex angles of a kite are .ASSESSMENT RESOURCESDG4CS 898 C.qxd8. A convex quadrilateral with congruent diagonals is a(n)or a(n) .9. An equilateral quadrilateral is a .10. The measure of an exterior angle of a regular octagon is.Part BFind each lettered angle measure.1. a 88 2. b 3. c 4. d 5. e 6. f 7. g 8. h d40 e70 bfgc76 h58 aPart CFind each lettered measure.1. Perimeter 64a 96 y10xx y 2. a yb a47 38bx y a24 x31(continued)Discovering Geometry Assessment ResourcesCHAPTER 571 2008 Key Curriculum PressDISCOVERING GEOMETRY COURSE SAMPLER141
DG4CS 898 C.qxd11/14/0611:23 AMPage 142Chapter 5 Test (continued)Form ANamePeriod3. a axw 31x y Dateyw60 55 464. A regular hexagonal mirror frame is to be built from stripsof 2-inch-wide pine lattice. At what angles a and b shouldthe lattice be cut?a bba ab ASSESSMENT RESOURCESPart DUse the segments and angle at right toconstruct each figure. Use either a compassand a straightedge or patty paper.xyz1. Rhombus WAVY using W and using . (You don’tsegment z as the diagonal WVneed to use segments x and y.)2. Kite LMNO using segments y and z asdiagonals and segment x as a side.(You don’t need to use W.)WPart EWrite a paragraph proof or a flowchart proof for the conjecture:The diagonals of a rectangle are congruent. and BD Given: Rectangle ABCD with diagonals AC BD Show: ACBCAD(continued)72CHAPTER 5Discovering Geometry Assessment Resources 2008 Key Curriculum Press142DISCOVERING GEOMETRY COURSE SAMPLER
11/14/0611:23 AMPage 143Chapter 5 Test (continued)Form ANamePeriodDateMixed Review CD and AD BC . Is AB 1. In the figure at right, AB ? Explain.parallel to CDBACDFor Problems 2 and 3, consider the statement: If two sides and an angle ofone triangle are congruent to two sides and an angle of another triangle,then the triangles are congruent.2. Is the statement true? If not, give a counterexample or explain why itis not true.ASSESSMENT RESOURCESDG4CS 898 C.qxd3. Write the converse of the statement. Is the converse true? If not, give acounterexample or explain why it is not true.4. Find x.45 xIn Problems 5–9, use the figure at right to name an example ofeach of these:Circle OAD5. An inscribed triangle6. An isosceles triangle7. A concave polygonBO8. An acute triangle9. A central angleFor Problems 10–12, m K 36 .C10. What is the measure of the complement to K ?11. What is the measure of the supplement to K ?12. What is the reflex measure of K ?Discovering Geometry Assessment ResourcesCHAPTER 573 2008 Key Curriculum PressDISCOVERING GEOMETRY COURSE SAMPLER143
DG4CS 898 C.qxd11/14/0611:23 AMPage 144Chapter 5 Constructive Assessment OptionsChoose one or more of these items to replace part of the chapter test.Let students know that they will receive from 0 to 5 points for eachitem, depending on the correctness and completeness of their answer.1. (Lesson 5.1)On her Chapter 5 test, Ms. Donovan asked her students to find themeasure of each interior angle of a regular 15-gon. Here are a few ofthe answers students gave:2340 156 180 24 168 a. Tell which of the answers is correct and explain why it is correct.b. Choose two of the incorrect answers and explain the error thestudent may have made in finding the answer.2. (Lessons 5.1–5.2)ASSESSMENT RESOURCESIn Lesson 5.1, you found formulas for the sum of the interior anglemeasures of a regular polygon and for the measure of each interiorangle. Now you will consider the “outside” angles of a regular polygon.In the regular hexagon below, one “outside” angle is marked. Themeasure of this angle is the number of degrees in the rotationindicated by the arrow.Find a formula for the sum of the measures of the “outside” angles of aregular n-gon and a formula for the measure of one “outside” angle.Show and explain all your work and simplify the formulas as much aspossible.(continued)Discovering Geometry Assessment Resources 2008 Key Curriculum Press144DISCOVERING GEOMETRY COURSE SAMPLERCHAPTER 577
11/14/0611:23 AMPage 145Chapter 5 Constructive Assessment Options (continued)3. (Lessons 5.1–5.3)A classical semicircular arch is really half of a regular polygonbuilt with blocks whose faces are congruent isosceles trapezoids.For example, the inner arch in the diagram below is half of aregular 18-gon.KeystoneVoussoirAbutmentRiseSpanThe angle measures of the isosceles trapezoids forming the arch dependon how many blocks are used to build the arch.a. If a semicircular arch has only three blocks, what are the anglemeasures of the isosceles trapezoids? If an arch has five blocks,what are the angle measures? Show all your work.ASSESSMENT RESOURCESDG4CS 898 C.qxdb. Given the number of blocks, b, in the arch, find formulas for themeasures of the trapezoid base angles. You should find twoformulas—one for the “outer” base angles (those whose vertices areon the outside of the arch) and one for the “inner” base angles.Explain the reasoning you used to find the formulas.4. (Lessons 5.3, 5.5, and 5.6)In the game Find the Oddball, a player looks at four objects anddetermines which one doesn’t belong with the others. For example,consider the four objects below.A.B.C.D.You might say that object B doesn’t belong because it has four sides,while the other shapes each have three. Or, you might say that object Cdoesn’t belong because it is the only shape with a right angle. You maybe able to find and explain other oddballs.Create three different groups of four quadrilaterals, each with at leastone shape that can be considered an oddball. For each group youcreate, identify every possible oddball and explain why it doesn’tbelong with the other objects.(continued)78CHAPTER 5Discovering Geometry Assessment Resources 2008 Key Curriculum PressDISCOVERING GEOMETRY COURSE SAMPLER145
DG4CS 898 C.qxd11/14/0611:23 AMPage 146Chapter 5 Constructive Assessment Options (continued)5. (Lessons 5.3, 5.5, and 5.6)Consider the following points: A(1, 4), B(2, 12), C(9, 8).a. Graph the points. Add a fourth point D so that points A, B, C, andD are the vertices of a particular type of quadrilateral. Name thequadrilateral and use algebra to verify one of the properties of thattype of quadrilateral. Show all your work.b. Find the coordinates of the point where the diagonals ofquadrilateral ABCD intersect. Show all your work.6. (Lesson 5.4)The modern art section of the Museum of Geometric Art is a largerectangular room. The museum directors want to build a wall in thecenter of the room to create more room for displaying art. The wallwill be built so that it is parallel to two of the opposite sides and itsends are equally distant from the other two sides.ASSESSMENT RESOURCESOnce the center wall is in place, a path will be paintedon the floor around it. The path will be created byconnecting the midsegments of the triangles and trapezoidsformed by connecting the ends of the center wall to thecorners of the room. (See the diagram.)PathCenter walla. If the room measures 80 ft by 100 ft and the wall is 70 ftlong, how long will the path be? Does your answerdepend on which sides the wall is parallel to? Explain.b. Now generalize your results. If the room measures a feetby b feet and the center wall is x feet long, how long willthe path around the wall be?Discovering Geometry Assessment Resources 2008 Key Curriculum Press146DISCOVERING GEOMETRY COURSE SAMPLERCHAPTER 579
11/14/0611:23 AMPage 147Lesson 5.1 Polygon Sum ConjectureNamePeriodDateIn Exercises 1 and 2, find each lettered angle measure.1. a , b , c ,d , e 2. a , b , c ,d , e , f eded 97 fabb26 c85 44 caP R AC T I C E YO U R S K I L L S W I T H A N S W E R SDG4CS 898 C.qxd3. One exterior angle of a regular polygon measures 10 . What isthe measure of each interior angle? How many sides does thepolygon have?4. The sum of the measures of the interior angles of a regular polygon is2340 . How many sides does the polygon have?5. ABCD is a square. ABE is an equilateral6. ABCDE is a regular pentagon. ABFGtriangle.is a square.x x DCDExEGFCxBABA7. Use a protractor to draw pentagon ABCDE with m A 85 ,m B 125 , m C 110 , and m D 70 . What is m E?Measure it, and check your work by calculating.32CHAPTER 5Discovering Geometry Practice Your Skills 2008 Key Curriculum PressDISCOVERING GEOMETRY COURSE SAMPLER147
DG4CS 898 C.qxd11/14/0611:23 AMPage 148Lesson 5.4 Properties of MidsegmentsNamePeriodDateIn Exercises 1–3, each figure shows a midsegment.1. a , b ,2. x , y ,c 3. x , y ,z z 41b1614zy54 ac13x37 y29z11x21P R AC T I C E YO U R S K I L L S W I T H A N S W E R S4. X, Y, and Z are midpoints. Perimeter PQR 132, RQ 55, and PZ 20.Perimeter XYZ RZPQ ZX P is the midsegment. Find the5. MNYQX6. Explain how to find the width of the lake fromcoordinates of M and N. Find the and MN .slopes of AByA to B using a tape measure, but withoutusing a boat or getting your feet wet.C (20, 10)BALakeMA (4, 2)NxB (9, –6)7. M, N, and O are midpoints. What type of quadrilateralCis AMNO? How do you know? Give a flowchart proofshowing that ONC MBN.NOAMB8. Give a paragraph or flowchart proof.Given: PQR with PD DF FH HRRand QE EG GI IRH FG DE PQ Show: HIFDPDiscovering Geometry Practice Your Skills 2008 Key Curriculum Press148DISCOVERING GEOMETRY COURSE SAMPLERIGEQCHAPTER 535
11/14/0611:23 AMPage 149CONDENSEDLESSON5.1Polygon Sum ConjectureIn this lesson you will Discover a formula for finding the sum of the angle measures for any polygonUse deductive reasoning to explain why the polygon sum formula worksTriangles come in many different shapes and sizes. However, as you discovered inChapter 4, the sum of the angle measures of any triangle is 180 . In this lessonyou will investigate the sum of the angle measures of other polygons. After youfind a pattern, you’ll write a formula that relates the number of sides of a polygonto the sum of the measures of its angles.C O N D E N S E D L E S S O N S : A T O O L F O R PA R E N T S A N D T U T O R SDG4CS 898 C.qxdInvestigation: Is There a Polygon Sum Formula?Draw three different quadrilaterals. For each quadrilateral, carefully measure thefour angles and then find the sum of the angle measures. If you measurecarefully, you should find that all of your quadrilaterals have the same angle sum.What is the sum? Record it in a table like the one below.Number of sidesSum of angle measures3456180 78900 1080 .nNext draw three different pentagons. Carefully measure the angles in eachpentagon and find the angle sum. Again, you should find that the angle sum isthe same for each pentagon. Record the sum in the table.Use your findings to complete the conjectures below.Quadrilateral Sum Conjecture The sum of the measures of the four anglesof any quadrilateral is .C-29Pentagon Sum Conjecture The sum of the measures of the five angles ofany pentagon is .C-30Now draw at least two different hexagons and find their angle sum. Record thesum in the table.The angle sums for heptagons and octagons have been entered in the table foryou, but you can check the sums by drawing and measuring your own polygons.Look for a pattern in the completed table. Find a general formula for the sum ofthe angle measures of a polygon in terms of the number of sides, n. (Hint: Usewhat you learned in Chapter 2 about finding the formula for a pattern with aconstant difference.) Then complete this conjecture.Polygon Sum Conjecture The sum of the measures of the n interior anglesof an n-gon is .C-31(continued)Discovering Geometry Condensed LessonsCHAPTER 565 2008 Key Curriculum PressDISCOVERING GEOMETRY COURSE SAMPLER149
DG4CS 898 C.qxd11/14/0611:23 AMPage 150Lesson 5.1 Polygon Sum Conjecture (continued)You can use deductive reasoning to see why your formula works. In each polygonbelow, all the diagonals from one vertex have been drawn, creating triangles.Notice that in each polygon, the number of triangles is 2 less than the numberof sides.C O N D E N S
98 CHAPTER 5 Discovering Geometry Solutions Manual 2008 Key Curriculum Press SOLUTIONS MANUAL 132 DISCOVERING GEOMETRY COURSE SAMPLER DG4CS_898_C.qxd 11/14/06 11:23 AM Page 132. y x (2 1) 1 x y 1 2 1 y 1 1(x 2) y 1 x 2