Transcription
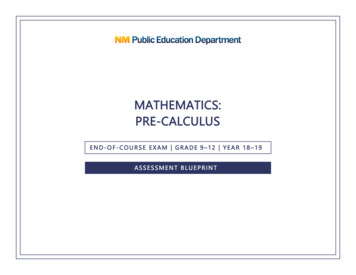
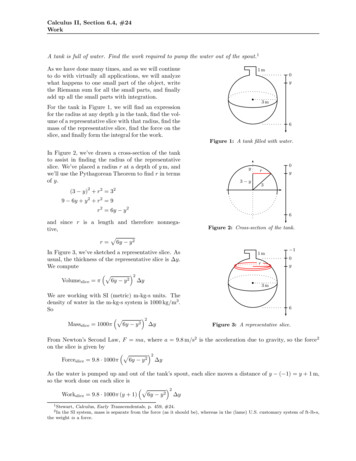
Calculus II, Section 6.4, #24WorkA tank is full of water. Find the work required to pump the water out of the spout.1As we have done many times, and as we will continueto do with virtually all applications, we will analyzewhat happens to one small part of the object, writethe Riemann sum for all the small parts, and finallyadd up all the small parts with integration.1m0y3mFor the tank in Figure 1, we will find an expressionfor the radius at any depth y in the tank, find the volume of a representative slice with that radius, find themass of the representative slice, find the force on theslice, and finally form the integral for the work.6Figure 1: A tank filled with water.In Figure 2, we’ve drawn a cross-section of the tankto assist in finding the radius of the representativeslice. We’ve placed a radius r at a depth of y m, andwe’ll use the Pythagorean Theorem to find r in termsof y.22(3 y) r 3y0r3 yy329 6y y 2 r2 9r2 6y y 2and since r is a length and therefore nonnegative,!r 6y y 2In Figure 3, we’ve sketched a representative slice. Asusual, the thickness of the representative slice is y.We compute#2"!6y y 2 yVolumeslice πWe are working with SI (metric) m-kg-s units. Thedensity of water in the m-kg-s system is 1000 kg/m3.So"!#2Massslice 1000π6y y 2 y6Figure 2: Cross-section of the tank.1m 10ry3m6Figure 3: A representative slice.From Newton’s Second Law, F ma, where a 9.8 m/s2 is the acceleration due to gravity, so the force2on the slice is given by"!#2Forceslice 9.8 · 1000π6y y 2 yAs the water is pumped up and out of the tank’s spout, each slice moves a distance of y ( 1) y 1 m,so the work done on each slice is#2"!Workslice 9.8 · 1000π (y 1)6y y 2 y1 Stewart,Calculus, Early Transcendentals, p. 459, #24.the SI system, mass is separate from the force (as it should be), whereas in the (lame) U.S. customary system of ft-lb-s,the weight is a force.2 In
Calculus IIWorkWe add up all the slices and take the limit as the number of slices increases without bound. The limit ofthe Riemann sum isW limn n i 1%&9800π (y 1) 6y y 2 yWe create slices from y 0 to y 6 (there are no slices in the spout), so we getW 'y 6y 0&%9800π (y 1) 6y y 2 dy 9800π' 9800π'y 66y 2 y 3 6y y 2 dyy 0y 6 y 3 5y 2 6y dyy 0)y 6y 4 5y 32 9800π 3y43y 0 * 4 )(* 435·605 · 036 3 · 62 3 · 02 9800π 4343()1296 1080 9800π 108 0 0 043 9800π · 144( 4,433,416or 4.4 106Thus, the amount of work to pump all the water out of this tank is about 4.4 106 Newton · m or about4.4 106 J.In our solution to this problem, we placed y 0 at the top of the spherical portion of the tank, and y 6at the bottom. Students often ask about the “best” placement for the coordinates, and the honest answer isthat it just doesn’t matter. As long as we are consistent with the algebraic expressions that arise from thegeometry, the end result will be the same. On the following pages, we’ll set up the problem three additionalways, and yet the value for the work will be the same each time.33 Yes,my fingers were crossed as I typed that sentence. It did not make the typing any easier.
Calculus II Work We add up all the slices and take the limit as the number of slices increases without bound. The limit of the Riemann sum is W lim