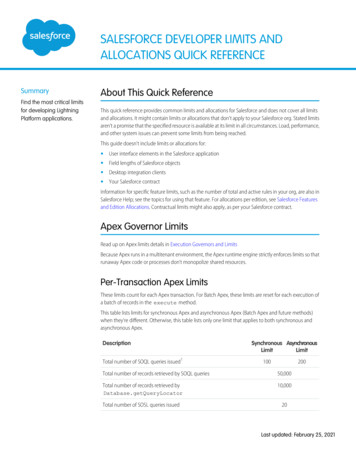
Transcription
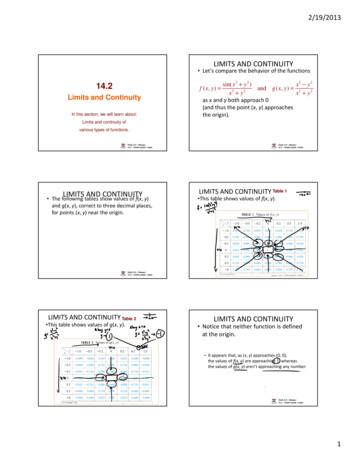
2/19/2013LIMITS AND CONTINUITY Let’s compare the behavior of the functions14.2sin( x 2 y 2 )x2 y 2andg(x,y) x2 y2x2 y2as x and y both approach 0(and thus the point (x, y) approachesthe origin).f ( x, y ) Limits and ContinuityIn this section, we will learn about:Limits and continuity ofvarious types of functions.Math 114 – Rimmer14.2 – Multivariable Limits LIMITS AND CONTINUITYThe following tables show values of f(x, y)Math 114 – Rimmer14.2 – Multivariable LimitsLIMITS AND CONTINUITY Table 1 This table shows values of f(x, y).and g(x, y), correct to three decimal places,for points (x, y) near the origin.Math 114 – Rimmer14.2 – Multivariable LimitsLIMITS AND CONTINUITY Table 2 This table shows values of g(x, y).Math 114 – Rimmer14.2 – Multivariable LimitsLIMITS AND CONTINUITY Notice that neither function is definedat the origin.– It appears that, as (x, y) approaches (0, 0),the values of f(x, y) are approaching 1, whereasthe values of g(x, y) aren’t approaching any number.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable Limits1
2/19/2013LIMITS AND CONTINUITY It turns out that these guesses based onnumerical evidence are correct. Thus, we write:LIMITS AND CONTINUITY In general, we use the notationlim( x , y ) ( a ,b )f ( x, y ) Lto indicate that:sin( x 2 y 2 ) 1– ( x , y ) (0,0)x2 y2lim2–lim( x , y ) (0,0)– The values of f(x, y) approach the number Las the point (x, y) approaches the point (a, b)along any path that stays within the domain of f.2x yx2 y2does not exist.Math 114 – Rimmer14.2 – Multivariable LimitsLIMITS AND CONTINUITY In other words, we can make the valuesof f(x, y) as close to L as we like by takingthe point (x, y) sufficiently close to the point(a, b), but not equal to (a, b).Math 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTIONDefinition 1 Let f be a function of two variableswhose domain D includes points arbitrarily closeto (a, b). Then, we say that the limit of f(x, y)as (x, y) approaches (a, b) is L.Math 114 – Rimmer14.2 – Multivariable LimitsSINGLE VARIABLE FUNCTIONS For functions of a single variable, when welet x approach a, there are only two possibledirections of approach, from the left or from theright.Math 114 – Rimmer14.2 – Multivariable LimitsDOUBLE VARIABLE FUNCTIONS For functions of twovariables, the situationis not as simple.– We recall from Chapter 2 that, ifthen lim f ( x) does not exist. lim f ( x ) lim f ( x),x ax ax aMath 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable Limits2
2/19/2013DOUBLE VARIABLE FUNCTIONS This is because we can let (x, y) approach(a, b) from an infinite number of directionsin any manner whatsoever as long as (x, y) stayswithin the domain of f.LIMIT OF A FUNCTION Definition 1 refers only to the distancebetween (x, y) and (a, b).– It does not refer to the direction of approach.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTION Therefore, if the limit exists, then f(x, y) mustapproach the same limit no matter how (x, y)approaches (a, b).LIMIT OF A FUNCTION Thus, if we can find two different paths ofapproach along which the function f(x, y)has different limits, then it follows thatdoes not exist.lim( x , y ) ( a ,b )f ( x, y )Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTION Iff(x, y) L1 as (x, y) (a, b) along a path C1 andf(x, y) L2 as (x, y) (a, b) along a path C2,where L1 L2,thenlim( x , y ) ( a ,b )LIMIT OF A FUNCTIONExample 1 Show thatx2 y2( x , y ) (0,0) x 2 y 2limdoes not exist.f ( x, y )– Let f(x, y) (x2 – y2)/(x2 y2).does not exist.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable Limits3
2/19/2013LIMIT OF A FUNCTIONLIMIT OF A FUNCTIONExample 1Example 1 First, let’s approach (0, 0) alongthe x-axis. We now approach along the y-axis byputting x 0.– Then, y 0 gives f(x, 0) x2/x2 1 for all x 0.– Then, f(0, y) –y2/y2 –1 for all y 0.– So, f(x, y) 1 as (x, y) (0, 0) along the x-axis.– So, f(x, y) –1 as (x, y) (0, 0) along the y-axis.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTION Since f has two different limits alongtwo different lines, the given limit doesnot exist.– This confirmsthe conjecture wemade on the basisof numerical evidenceat the beginningof the section.LIMIT OF A FUNCTIONExample 2 Iff ( x, y ) xyx y2limf ( x, y )Example 1does( x , y ) (0,0)2exist?Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTIONExample 2LIMIT OF A FUNCTIONExample 2 If y 0, then f(x, 0) 0/x2 0.– Therefore, If x 0, then f(0, y) 0/y2 0.– So,f(x, y) 0 as (x, y) (0, 0) along the x-axis.Math 114 – Rimmer14.2 – Multivariable Limitsf(x, y) 0 as (x, y) (0, 0) along the y-axis.Math 114 – Rimmer14.2 – Multivariable Limits4
2/19/2013LIMIT OF A FUNCTIONLIMIT OF A FUNCTIONExample 2Example 2 Although we have obtained identical limitsalong the axes, that does not show thatthe given limit is 0. Let’s now approach (0, 0) along anotherline, say y x.– For all x 0,f ( x, x ) – Therefore,x21 x x2 22f ( x, y ) 12 as ( x, y ) (0, 0) along y xMath 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTIONExample 2 Since we have obtained different limitsalong different paths, the given limit doesnot exist.Math 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTION This figure sheds somelight onExample 2.– The ridge that occursabove the line y xcorresponds to the factthat f(x, y) ½ for allpoints (x, y) on that lineexcept the origin.Math 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTIONMath 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTIONExample 3 IfdoesExample 3xy 2f ( x, y ) 2x y4lim( x , y ) (0,0) With the solution of Example 2 in mind,let’s try to save time by letting (x, y) (0, 0)along any nonvertical line through the origin.f ( x, y )exist?Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable Limits5
2/19/2013LIMIT OF A FUNCTIONLIMIT OF A FUNCTIONExample 3Example 3 Then, y mx, where m is the slope,andf ( x, y ) f ( x, mx) Therefore,f(x, y) 0 as (x, y) (0, 0) along y mxx(mx) 2x 2 (mx) 4m2 x3x 2 m4 x 4m2 x 1 m4 x2– Thus, f has the same limiting value alongevery nonvertical line through the origin. Math 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTIONMath 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTIONExample 3 However, that does not show thatthe given limit is 0.Example 3– This is because, if we now let(x, y) (0, 0) along the parabola x y2we have:y2 y2y42f ( x, y ) f ( y , y ) 2 2(y ) y4 2y4 12 Since different paths lead to differentlimiting values, the given limit does notexist.– So,f(x, y) ½ as (x, y) (0, 0) along x y2Math 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTION Now, let’s look at limitsthat do exist.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTION Just as for functions of one variable,the calculation of limits for functions oftwo variables can be greatly simplifiedby the use of properties of limits.Math 114 – Rimmer14.2 – Multivariable Limits6
2/19/2013LIMIT OF A FUNCTION The Limit Laws listed in Section 2.3 can beextended to functions of two variables. For instance,LIMIT OF A FUNCTIONEquations 2 In particular, the following equationsare true.limx alimy blimc c( x , y ) ( a ,b )– The limit of a sum is the sum of the limits.( x , y ) ( a ,b )– The limit of a product is the product of the limits.Math 114 – Rimmer14.2 – Multivariable LimitsLIMIT OF A FUNCTIONEquations 2( x , y ) ( a ,b )Math 114 – Rimmer14.2 – Multivariable LimitsCONTINUITY OF SINGLE VARIABLEFUNCTIONS Recall that evaluating limits of continuousfunctions of a single variable is easy. The Squeeze Theoremalso holds.– It can be accomplished by direct substitution.– This is because the defining property ofa continuous function islim f ( x ) f (a )x aMath 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable Limits7
2/19/2013CONTINUITY OF DOUBLE VARIABLEFUNCTIONS Continuous functions of two variablesare also defined by the direct substitutionproperty.Math 114 – Rimmer14.2 – Multivariable LimitsCONTINUITYCONTINUITYDefinition 4 A function f of two variables is called continuousat (a, b) iflim( x , y ) ( a ,b )Math 114 – Rimmer14.2 – Multivariable Limitsf ( x, y ) f ( a, b ) We say f is continuous on D if f iscontinuous at every point (a, b) in D. The intuitive meaning of continuity is that,if the point (x, y) changes by a small amount,then the value of f(x, y) changes by a smallamount.– This means that a surface that is the graph ofa continuous function has no hole or break.Math 114 – Rimmer14.2 – Multivariable LimitsCONTINUITYMath 114 – Rimmer14.2 – Multivariable LimitsPOLYNOMIAL Using the properties of limits, you can seethat sums, differences, products, quotientsof continuous functions are continuous on theirdomains.– Let’s use this fact to give examplesof continuous functions. A polynomial function of two variables(polynomial, for short) is a sum of termsof the form cxmyn,where:– c is a constant.– m and n are nonnegative integers.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable Limits8
2/19/2013RATIONAL FUNCTIONRATIONAL FUNCTION VS. POLYNOMIALf ( x, y ) x 4 5 x 3 y 2 6 xy 4 7 y 6 A rational function isa ratio of polynomials. is a polynomial.g ( x, y ) 2 xy 1x2 y2 is a rational function.Math 114 – Rimmer14.2 – Multivariable LimitsCONTINUITYMath 114 – Rimmer14.2 – Multivariable LimitsCONTINUOUS POLYNOMIALS The limits in Equations 2 show thatthe functions Any polynomial can be built up outof the simple functions f, g, and hby multiplication and addition.f(x, y) x, g(x, y) y, h(x, y) c– It follows that all polynomials are continuouson R2.are continuous.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable LimitsCONTINUOUS RATIONALFUNCTIONSCONTINUITYExample 5 Likewise, any rational function is continuouson its domain because it isa quotient of continuous functions. Evaluatelim ( x 2 y 3 x 3 y 2 3 x 2 y )( x , y ) (1,2)2 33 2– f ( x, y ) x y x y 3 x 2 y is a polynomial.– Thus, it is continuous everywhere.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable Limits9
2/19/2013CONTINUITYCONTINUITYExample 5Example 6– Hence, we can find the limit by directsubstitution: Where is the functionlim ( x 2 y 3 x3 y 2 3x 2 y )( x , y ) (1,2)233continuous?x2 y 2f ( x, y ) 2x y22 1 2 1 2 3 1 2 2 11Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable LimitsCONTINUITYCONTINUITYExample 6Example 7 The function f is discontinuous at (0, 0)because it is not defined there. Let Since f is a rational function, it is continuous onits domain, which is the setD {(x, y) (x, y) (0, 0)} x2 y2if ( x, y ) (0, 0) 2g ( x, y ) x y 2 0if ( x, y ) (0, 0) – Here, g is defined at (0, 0).– However, it is still discontinuous there becauselim( x , y ) (0,0)g ( x, y )does not exist (see Example 1).Math 114 – Rimmer14.2 – Multivariable LimitsCONTINUITYMath 114 – Rimmer14.2 – Multivariable LimitsCOMPOSITE FUNCTIONS Just as for functions of one variable,composition is another way of combiningtwo continuous functions to get a third. This figure shows thegraph ofthe continuous functionin Example 8.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable Limits10
2/19/2013COMPOSITE FUNCTIONS In fact, it can be shown that, if f isa continuous function of two variables andg is a continuous function of a single variabledefined on the range of f, then– The composite function h g f defined byh(x, y) g(f(x, y)) is also a continuous function.COMPOSITE FUNCTIONSExample 9 Where is the function h(x, y) arctan(y/x)continuous?– The function f(x, y) y/x is a rational functionand therefore continuous except on the line x 0.– The function g(t) arctan t is continuous everywhere.Math 114 – Rimmer14.2 – Multivariable LimitsCOMPOSITE FUNCTIONSExample 9–So, the composite functionMath 114 – Rimmer14.2 – Multivariable LimitsCOMPOSITE FUNCTIONS Example 9 The figure shows thebreak in the graphof h above the y-axis.g(f(x, y)) arctan(y/ x) h(x, y)is continuous except where x 0.Math 114 – Rimmer14.2 – Multivariable LimitsMath 114 – Rimmer14.2 – Multivariable Limits11
14.2 – Multivariable Limits LIMITS AND CONTINUITY This table shows values of f(x, y). Table 1 Math 114 – Rimmer 14.2 – Multivariable Limits LIMITS AND CONTINUITY This table shows values of g(x, y). Table 2 Math 114 – Rimmer 14.2 – Multivariable Limits LIMITS AND CONTINUITY