WIXLIB

Solutions toTopologyChapter 2 - Topological Spaces and Continuous FunctionsJames MunkresSolutions by positrón0802https://positron0802.wordpress.com1 January 2021Contents2 Topological Spaces and Continuous Functions12 Topological Spaces . . . . . . . . . . . . . .13 Basis for a Topology . . . . . . . . . . . . . .14 The Order Topology . . . . . . . . . . . . . .15 The Product Topology on 𝑋 𝑌 . . . . . . .16 The Subspace Topology . . . . . . . . . . . .17 Closed Sets and Limit Points . . . . . . . . .18 Continuous Functions . . . . . . . . . . . . .19 The Product Topology . . . . . . . . . . . . .20 The Metric Topology . . . . . . . . . . . . .21 The Metric Topology (continued) . . . . . .22 The Quotient Topology . . . . . . . . . . . .Supplementary Exercises: Topological Groups . .2Topological Spaces and Continuous Functions12Topological Spaces.1114446111417232728No Exercises.13Basis for a TopologyExercise 13.1. For every 𝑥 𝐴 there is an open set 𝑈𝑥 such that 𝑥 𝑈𝑥 and 𝑈𝑥 𝐴. ThenÐ𝐴 𝑥 𝐴 𝑈𝑥 is open in 𝑋 since it is a union of open sets.1

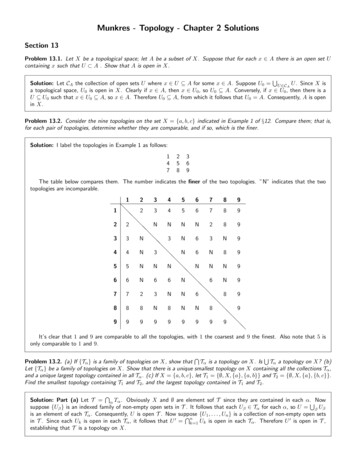
13Basis for a TopologyExercise 13.2. These topologies are listed below:𝒯1 { , 𝑋 },𝒯2 { , {𝑎}, {𝑎, 𝑏}, 𝑋 },𝒯3 { , {𝑏}, {𝑎, 𝑏}, {𝑏, 𝑐}, 𝑋 },𝒯4 { , {𝑏}, 𝑋 },𝒯5 { , {𝑎}, {𝑏, 𝑐}, 𝑋 },𝒯6 { , {𝑏}, {𝑐}, {𝑎, 𝑏}, {𝑏, 𝑐}, 𝑋 },𝒯7 { , {𝑎, 𝑏}, 𝑋 },𝒯8 { , {𝑎}, {𝑏}, {𝑎, 𝑏}, 𝑋 },𝒯9 { , {𝑎}, {𝑏}, {𝑐}, {𝑎, 𝑏}, {𝑎, 𝑐}, {𝑏, 𝑐}, 𝑋 }.𝒯1 is coarser than any other topology, and 𝒯9 is finer than any other topology. Other inclusionsare 𝒯7 𝒯2 𝒯8, 𝒯4 𝒯3 𝒯6, 𝒯7 𝒯3 𝒯6 and 𝒯4 𝒯8 .Exercise 13.3. 𝒯𝑐 is the collection of all subsets 𝑈 of 𝑋 such that 𝑋 \ 𝑈 is either countable or isall of 𝑋 . Clearly and 𝑋 are both in 𝒯𝑐 . Let {𝑈𝛼 }𝛼 𝐽 be a family of sets in 𝒯𝑐 . ThenØÙ𝑋\𝑈𝛼 (𝑋 \ 𝑈𝛼 )𝛼 𝐽𝛼 𝐽is an intersection of countable sets, hence countable. If 𝑈 1, . . . , 𝑈𝑛 are elements in 𝒯𝑐 , then𝑋\𝑛Ù𝑈𝑖 𝑖 1𝑛Ø(𝑋 \ 𝑈𝑖 )𝑖 1is countable being a union of countable sets. It follows that 𝒯𝑐 is a topology on 𝑋 .On the other hand, 𝒯 is in general not a topology on 𝑋 . For example, let 𝑋 R, 𝑈 1 ( , 0)and 𝑈 2 (0, ). Then 𝑈 1 and 𝑈 2 are in 𝒯 but 𝑈 1 𝑈 2 R \ {0} is not.ÑExercise 13.4. (a) Since and 𝑋 belong to 𝒯𝛼 for each 𝛼, they belong to 𝛼 𝒯𝛼 . Let {𝑉𝛽 }𝛽 beÑÐa collection of open sets in 𝛼 𝒯𝛼 . For any fixed 𝛼 we have 𝛽 𝑉𝛽 𝒯𝛼 since 𝒯𝛼 is a topologyÐÑÑon 𝑋, so 𝛽 𝑉𝛽 𝛼 𝒯𝛼 . Similarly, if 𝑈 1, . . . , 𝑈𝑛 are elements of 𝛼 𝒯𝛼 , then for each 𝛼 we haveÑÑÐ𝑛Ð𝑛 𝛼 𝒯𝛼 . It follows that 𝛼 𝒯𝛼 is a topology on 𝑋 .𝑖 1 𝑈𝑖 𝒯𝛼 and therefore 𝑖 1 𝑈𝑖 ÐOn the other hand, the union 𝛼 𝒯𝛼 is in general not a topology on 𝑋 . For instance, let𝑋 {𝑎, 𝑏, 𝑐}. Then 𝒯1 { , 𝑋, {𝑎}} and 𝒯2 { , 𝑋, {𝑏}} are topologies on 𝑋 but 𝒯1 𝒯2 { , 𝑋, {𝑎}, {𝑏}} is not.(b) First we prove that there is a unique smallest topology on 𝑋 containing all the collections𝒯𝛼 . Uniqueness of such topology is clear. For each 𝛼 let ℬ𝛼 be a basis for 𝒯𝛼 . Let 𝒯 be theÐtopology generated by the subbasis 𝒮 𝛼 ℬ𝛼 . Then the collection ℬ of all finite intersectionsof elements of 𝒮 is a basis for 𝒯. Clearly 𝒯𝛼 𝒯 for all 𝛼 . We now prove that if 𝒪 is a topologyon 𝑋 such that 𝒯𝛼 𝒪 for all 𝛼, then 𝒯 𝒪. Given such 𝒪, we have ℬ𝛼 𝒪 for all 𝛼, so 𝒮 𝒪.Since 𝒪 is a topology, it must contain all finite intersections of elements of 𝒮, so ℬ 𝒪 and henceÐ𝒯 𝒪. We conclude that the topology 𝒯 generated by the subbasis 𝒮 𝛼 ℬ𝛼 is the uniquesmallest topology on 𝑋 containing all the collections 𝒯𝛼 .Now we prove that there exists a unique largest topology contained in all 𝒯𝛼 . Uniqueness ofÑsuch topology is clear. Consider 𝒯 𝛼 𝒯𝛼 . We already know that 𝒯 is a topology by (a), and2Solutions by positrón0802

13Basis for a Topologyclearly 𝒯 𝒯𝛼 for all 𝛼 . If 𝒪 is another topology contained in all 𝒯𝛼 , it must be contained intheir intersection, so 𝒪 𝒯. I follows that 𝒯 is the unique largest topology contained in all 𝒯𝛼 .(c) We use the results obtained in (b). The smallest topology containing 𝒯1 and 𝒯2 has 𝒯1 𝒯2 { , 𝑋, {𝑎}, {𝑎, 𝑏}, {𝑏, 𝑐}} as subbasis; taking unions of intersections of elements of 𝒯1 𝒯2 , wefound that this topology is { , 𝑋, {𝑎}, {𝑏}, {𝑎, 𝑏}, {𝑏, 𝑐}}. The largest topology contained in both𝒯1 and 𝒯2 is their intersection 𝒯1 𝒯2 { , 𝑋, {𝑎}}.Exercise 13.5. Let 𝒯 be the topology generated by 𝒜 and let 𝒪 be the intersection of all topologies on 𝑋 that contains 𝒜. Clearly 𝒪 𝒯 since 𝒯 is a topology on 𝑋 that contain 𝒜. Conversely,let 𝑈 𝒯, so that 𝑈 is a union of elements of 𝒜. Since each of this elements is also an elementof 𝒪, their union 𝑈 belongs to 𝒪. Thus 𝒯 𝒪 and the equality holds.If we now considered 𝒜 as a subbasis, then the elements of 𝒯 are union of finite intersectionsof elements of 𝒜. The inclusion 𝒪 𝒯 is again clear and 𝒯 𝒪 holds since every union of finiteintersections of elements of 𝒜 belongs to 𝒪.Exercise 13.6. Let 𝒯ℓ and 𝒯𝐾 denote the topologies of Rℓ and R𝐾 respectively. Given the basiselement [0, 1) for 𝒯ℓ , there is no basis element for 𝒯𝐾 containing 0 and contained in [0, 1), so𝒯ℓ 𝒯𝐾 . Similarly, given the basis element ( 1, 1) \ 𝐾 for 𝒯𝐾 , there is no basis element for 𝒯ℓcontaining 0 contained in ( 1, 1) \ 𝐾, so 𝒯𝐾 𝒯ℓ .Exercise 13.7. Given 𝑎 𝑏 in R, if 𝑥 (𝑎, 𝑏) \ 𝐾 then 𝑥 (𝑥 𝜀, 𝑥] (𝑎, 𝑏) \ 𝐾 for sufficientlysmall 𝜀. Thus 𝒯4 is finer than 𝒯2 . Every basis element for 𝒯5 is open in 𝒯1, and every basis elementfor 𝒯1 is a basis element for 𝒯2 . It follows that 𝒯5 𝒯1 𝒯2 𝒯4 . Since every open set in 𝒯3is open in 𝒯1, we also have 𝒯3 𝒯1 𝒯2 𝒯4 . Finally, we prove that 𝒯3 and 𝒯5 are notcomparable. Indeed, R \ {0, 1} is open in 𝒯3, but it is not a union of basis elements for 𝒯5, so𝒯3 𝒯5 . Conversely, ( , 0) is open in 𝒯5, and is clearly not open in 𝒯3, so 𝒯5 𝒯3 .Exercise 13.8. (a) First note that ℬ is a basis for a topology on R. This follows from the fact thatthe union of its elements is all of R and the intersection of two elements of ℬ is either emptyor another element of ℬ. Let 𝒯 be the standard topology on R. Clearly the topology generatedby ℬ is coarser than 𝒯. Let 𝑈 𝒯 and 𝑥 𝑈 . Then 𝑈 contains an open interval with centre𝑥 . Since the rationals are dense in R with the standard topology, there exists 𝑞 Q such that𝑥 (𝑥 𝑞, 𝑥 𝑞) 𝑈 . This proves that 𝒯 is coarser than the topology generated by ℬ. Weconclude that ℬ generates the standard topology on R.(b) 𝒞 is a basis for a topology on R since the union of its elements is R and the intersectionof two elements of 𝒞 is either empty or another element of 𝒞. Now consider [𝑟, 𝑠) where 𝑟 is anyirrational number and 𝑠 is any real number greater than 𝑟 . Then [𝑟, 𝑠) is a basis element for thetopology of Rℓ , but [𝑟, 𝑠) is not a union of elements of 𝒞. Indeed, suppose that [𝑟, 𝑠) 𝛼 [𝑎𝛼 , 𝑏𝛼 )for rationals 𝑎𝛼 , 𝑏𝛼 . Then 𝑟 [𝑎𝛼 , 𝑏𝛼 ) for some 𝛼 . Since 𝑟 is irrational we must have 𝑎𝛼 𝑟, butthen 𝑎𝛼 [𝑟, 𝑠), a contradiction. It follows that the topology generated by 𝒞 is strictly coarserthan the lower limit topology on R.3Solutions by positrón0802

14The Order Topology14The Order TopologyNo Exercises.15The Product Topology on 𝑋 𝑌No Exercises.16The Subspace TopologyExercise 16.1. Let 𝒯 be the topology 𝐴 inherits as a subspace of 𝑌 , and 𝒪 be the topology itinherits as a subspace of 𝑋 . A (standard) basis element for 𝒯 has the form 𝑈 𝐴 where 𝑈 is openin 𝑌, so is of the form (𝑌 𝑉 ) 𝐴 𝑉 𝐴 where 𝑉 is open in 𝑋 . Therefore every basis elementfor 𝒯 is also a basis element for 𝒪. Conversely, a (standard) basis element for 𝒪 have the form𝑊 𝐴 𝑊 𝑌 𝐴 where 𝑊 is open in 𝑋 . Since 𝑊 𝑌 is open in 𝑌 , this is a basis element for𝒯, so every basis element for 𝒪 is a basis element for 𝒯. It follows that 𝒯 𝒪.Exercise 16.2. Denote by 𝒯𝑦0 and 𝒯𝑦 these corresponding subspace topologies. Since every openset in 𝒯𝑦 have the form 𝐴 𝑌 with 𝐴 𝒯 𝒯 0, every open set in 𝒯𝑦 is open in 𝒯𝑦0 . Therefore𝒯𝑦0 is finer than 𝒯𝑦 . However, it may not be strictly finer. If for example 𝑌 has only one element,then 𝒯𝑦 and 𝒯𝑦0 are equal.Exercise 16.3. 𝐴 is open in R and 𝐴 𝑌 𝐴, so it is also open in 𝑌 .𝐵 is not open in R, but 𝐵 𝑈 𝑌 where 𝑈 ( 2, 12 ) ( 12 , 2) is open in R, so 𝐵 is open in 𝑌 .𝐶 and 𝐷 are not open in R nor in 𝑌 , as there is no basis (for 𝑌 or R) open set containing12 𝐶 𝐷 and contained in 𝐶 or 𝐷. ( 1 , 1 ) is open in R. Since 𝐸 𝑌 𝐸, it is also open in 𝑌 .Finally, 𝐸 (0, 1) \ { 𝑛1 }𝑛 Z 𝑛 1𝑛 1 𝑛Exercise 16.4. Let 𝑈 𝑉 be a (standard) basis element for 𝑋 𝑌 , so that 𝑈 is open in 𝑋 and 𝑉is open in 𝑌 . Then 𝜋 1 (𝑈 𝑉 ) 𝑈 is open in 𝑋 and 𝜋 2 (𝑈 𝑉 ) 𝑉 is open in 𝑌 . Since arbitraryÐÐmaps and unions satisfy 𝑓 ( 𝛼 𝑊𝛼 ) 𝛼 𝑓 (𝑊𝛼 ), it follows that 𝜋 1 and 𝜋 2 are open maps.Exercise 16.5. (a) Let 𝑈 be a (standard) basis open set in 𝑋 𝑌 , so that 𝑈 𝑉 𝑊 where 𝑉 isopen in 𝑋 and 𝑊 is open in 𝑌 . Since 𝒯 𝒯 0 and 𝒰 𝒰 0, then 𝑉 is open in 𝑋 0 and 𝑊 is openin 𝑌 0 . Thus every basis open set in 𝑋 𝑌 is open in 𝑋 0 𝑌 0 and hence the product topology on𝑋 0 𝑌 0 is finer that the product topology on 𝑋 𝑌 .(b) The converse does hold. Suppose that the product topology on 𝑋 0 𝑌 0 is finer than theproduct topology on 𝑋 𝑌 . Let 𝑈 be open in 𝑋 and 𝑉 be open in 𝑌 ; let 𝑎 𝑈 and 𝑏 𝑉 . Since𝑈 𝑉 is open in 𝑋 𝑌 , it is also open in 𝑋 0 𝑌 0, so there exist an open set 𝑈 0 of 𝑋 0 and an openset 𝑉 0 of 𝑌 0 such that 𝑎 𝑏 𝑈 0 𝑉 0 𝑈 𝑉 . It follows that 𝑎 𝑈 0 𝑈 and 𝑏 𝑉 0 𝑉 . Weconclude that 𝒯 𝒯 0 and 𝒰 𝒰 0 .Exercise 16.6. In Exercise 13.8 we already proved thatℬ {(𝑎, 𝑏) 𝑎 𝑏, 𝑎 and 𝑏 rational}4Solutions by positrón0802