Transcription
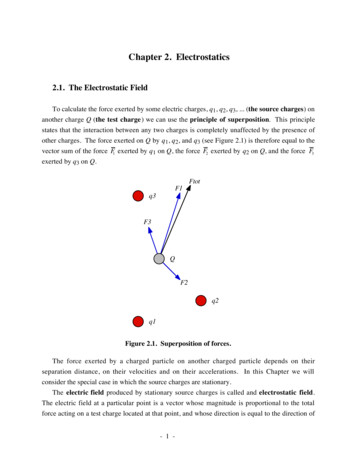
Chapter 2. Electrostatics2.1. The Electrostatic FieldTo calculate the force exerted by some electric charges, q1, q2, q3, . (the source charges) onanother charge Q (the test charge) we can use the principle of superposition. This principlestates that the interaction between any two charges is completely unaffected by the presence ofother charges. The force exerted on Q by q1, q2, and q3 (see Figure 2.1) is therefore equal to thevector sum of the force F1 exerted by q1 on Q, the force F2 exerted by q2 on Q, and the force F3exerted by q3 on Q.FtotF1q3F3QF2q2q1Figure 2.1. Superposition of forces.The force exerted by a charged particle on another charged particle depends on theirseparation distance, on their velocities and on their accelerations. In this Chapter we willconsider the special case in which the source charges are stationary.The electric field produced by stationary source charges is called and electrostatic field.The electric field at a particular point is a vector whose magnitude is proportional to the totalforce acting on a test charge located at that point, and whose direction is equal to the direction of- 1 -
the force acting on a positive test charge. The electric field E , generated by a collection ofsource charges, is defined asE FQwhere F is the total electric force exerted by the source charges on the test charge Q. It isassumed that the test charge Q is small and therefore does not change the distribution of thesource charges. The total force exerted by the source charges on the test charge is equal toˆ1 Ê q1Qq2Qq3QQ n qiF F1 F2 F3 . Á 2 rˆ 2 rˆ 2 rˆ . Â 2 rˆ4pe 0 Ë r1 1 r2 2 r3 3 4pe 0 i 1 ri iThe electric field generated by the source charges is thus equal toF1 n qiE Â 2 rˆQ 4pe 0 i 1 ri iIn most applications the source charges are not discrete, but are distributed continuously oversome region. The following three different distributions will be used in this course:1. line charge l: the charge per unit length.2. surface charge s: the charge per unit area.3. volume charge r: the charge per unit volume.To calculate the electric field at a point P generated by these charge distributions we have toreplace the summation over the discrete charges with an integration over the continuous chargedistribution:1. for a line charge: E( P ) 1rˆ2 l dlÚ4pe 0 Line r2. for a surface charge: E( P ) 1rˆ2 sdaÚ4pe 0 Surface r3. for a volume charge: E( P ) 1rˆ2 r dtÚ4pe 0 Volume r- 2 -
Here rˆ is the unit vector from a segment of the charge distribution to the point P at which weare evaluating the electric field, and r is the distance between this segment and point P .Example: Problem 2.2a) Find the electric field (magnitude and direction) a distance z above the midpoint between twoequal charges q a distance d apart. Check that your result is consistent with what you wouldexpect when z » d.b) Repeat part a), only this time make he right-hand charge -q instead of q.Etota)b)ErElElPErzd/2EtotPd/2d/2zd/2Figure 2.2. Problem 2.2a) Figure 2.2a shows that the x components of the electric fields generated by the two pointcharges cancel. The total electric field at P is equal to the sum of the z components of theelectric fields generated by the two point charges:E( P ) 21qˆ4pe 0 Ê 1 2d z2Ë4 z1 2d z24zˆ 1qzˆ3/ 2 z2pe 0 Ê 1 22ˆË4d z When z » d this equation becomes approximately equal toE( P ) @1 2q1 qˆ zˆ2 z4pe 0 z 22pe 0 z- 3 -
which is the Coulomb field generated by a point charge with charge 2q.b) For the electric fields generated by the point charges of the charge distribution shown inFigure 2.2b the z components cancel. The net electric field is therefore equal tod21qE( P ) 2ˆ4pe 0 Ê 1 2d z2Ë4 1 2d z24xˆ 1qdˆ3/ 2 x4pe 0 Ê 1 2ˆ2Ë4d z Example: Problem 2.5Find the electric field a distance z above the center of a circular loop of radius r which carriesa uniform line charge l.2dEzdErdElPzrFigure 2.3. Problem 2.5.Each segment of the loop is located at the same distance from P (see Figure 2.3). Themagnitude of the electric field at P due to a segment of the ring of length dl is equal todE 1l dl224pe 0 r z- 4 -
When we integrate over the whole ring, the horizontal components of the electric field cancel.We therefore only need to consider the vertical component of the electric field generated by eachsegment:dEz z2r z2dE l dlz3/ 224pe0 ( r z 2 )The total electric field generated by the ring can be obtained by integrating dEz over the wholering:E lz1z1z()dl 2prl 3/23/23/ 2 qÚ4pe 0 ( r 2 z 2 ) Ring4pe 0 ( r 2 z 2 )4pe0 ( r 2 z 2 )Example: Problem 2.7Find the electric field a distance z from the center of a spherical surface of radius R, whichcarries a uniform surface charge density s . Treat the case z R (inside) as well as z R(outside). Express your answer in terms of the total charge q on the surface.Pz-rcosqzqrcosqrsinqFigure 2.4. Problem 2.7.Consider a slice of the shell centered on the z axis (see Figure 2.4). The polar angle of thisslice is q and its width is dq. The area dA of this ring isdA (2p r sinq )rdq 2p r 2 sinqdqThe total charge on this ring is equal to- 5 -
dq sdA 1qsinqdq2where q is the total charge on the shell. The electric field produced by this ring at P can becalculated using the solution of Problem 2.5:dE 1 qz - r cosqr sinqdq8pe 0 r ( r 2 z 2 - 2zr cosq )3/ 2The total field at P can be found by integrating dE with respect to q:E 1 q pz - r cosqr sinqdq Ú8pe 0 r 0 ( r 2 z 2 - 2zr cosq )3/ 2 z - r cosq1 q rz -y1 q p()drcosq 3/23/ 2 dyÚÚ8pe 0 r -r ( r 2 z 2 - 2zy )8pe 0 r 0 ( r 2 z 2 - 2zr cosq )This integral can be solved using the following relation:z -y(r2 z 2 - 2zy )3/ 2 -ddz12r z 2 - 2zySubstituting this expression into the integral we obtain:1 q d1 q d r1dy E Ú8pe 0 r dz8pe 0 r dz -r r 2 z 2 - 2zy -r 2 z 2 - 2zy-z1 q d Ï( r z ) - r - z Ì8pe 0 r dz Óz Outside the shell, z r and consequently the electric field is equal toE -1 q d (r z ) - (z - r )1d 11 q q 28pe 0 r dzz4pe 0 dz z 4pe 0 zInside the shell, z r and consequently the electric field is equal toE -1 q d1 q d (r z ) - (r - z ) 1 0z4pe 0 r dz8pe 0 r dz- 6 -r -r
Thus the electric field of a charged shell is zero inside the shell. The electric field outside theshell is equal to the electric field of a point charge located at the center of the shell.2.2. Divergence and Curl of Electrostatic FieldsThe electric field can be graphically represented using field lines. The direction of the fieldlines indicates the direction in which a positive test charge moves when placed in this field. Thedensity of field lines per unit area is proportional to the strength of the electric field. Field linesoriginate on positive charges and terminate on negative charges. Field lines can never crosssince if this would occur, the direction of the electric field at that particular point would beundefined. Examples of field lines produced by positive point charges are shown in Figure 2.5.a)b)Figure 2.5. a) Electric field lines generated by a positive point charge with charge q. b)Electric field lines generated by a positive point charge with charge 2q.The flux of electric field lines through any surface is proportional to the number of field linespassing through that surface. Consider for example a point charge q located at the origin. Theelectric flux F E through a sphere of radius r, centered on the origin, is equal toFE ÚSurfaceE da 1qÊ q ˆˆ ( r 2 sinqdqdfrˆ ) 2 rÚe04pe 0 Surface Ë r Since the number of field lines generated by the charge q depends only on the magnitude of thecharge, any arbitrarily shaped surface that encloses q will intercept the same number of fieldlines. Therefore the electric flux through any surface that encloses the charge q is equal to q / e0 .Using the principle of superposition we can extend our conclusion easily to systems containingmore than one point charge:- 7 -
FE E da ÂÚiSurfaceÚEi da Surface1qie0 ÂiWe thus conclude that for an arbitrary surface and arbitrary charge distributionÚQenclosede0E da Surfacewhere Qenclosed is the total charge enclosed by the surface. This is called Gauss's law. Sincethis equation involves an integral it is also called Gauss's law in integral form.Using the divergence theorem the electric flux F E can be rewritten asÚFE Ú ( — E )dtE da SurfaceVolumeWe can also rewrite the enclosed charge Qencl in terms of the charge density r:Qenclosed Úr dtVolumeGauss's law can thus be rewritten asÚ ( — E )dt Volume1Ú rdte 0 VolumeSince we have not made any assumptions about the integration volume this equation must holdfor any volume. This requires that the integrands are equal:— E re0This equation is called Gauss's law in differential form.Gauss's law in differential form can also be obtained directly from Coulomb's law for acharge distribution r( r ') :E( r ') 1Dˆr2 r ( r ')dt 'Ú4pe 0 Volume ( Dr )where Dr r - r '. The divergence of E r is equal to- 8 -
— E( r ) Ê1Dˆr ˆ1r( r )— 4pd 3 ( r - r ')r ( r ')dt ' Á2 r (r ')dt ' ÚÚe04pe 0 Volume Ë4pe 0 Volume( Dr ) which is Gauss's law in differential form. Gauss's law in integral form can be obtained byintegrating — E( r ) over the volume V:Ú ( — E ( r ))dt ÚVolumeE da F E Surfacer( r )Qdt Enclosede0e0VolumeÚExample: Problem 2.42If the electric field in some region is given (in spherical coordinates) by the expressionE( r ) Aˆr B sinq cosffˆrwhere A and B are constants, what is the charge density r ?The charge density r can be obtained from the given electric field, using Gauss's law indifferential form:rÊ1 ˆ11 e0( — E ) e 0 Á 2 ( r 2 Er ) sinqEq ) Ef (Ë r rr sinq qr sinq f ( )Ê1 1 Ê B sinq cosf ˆ ˆAB e0 Á 2 ( Ar ) e- e 0 2 sin f 02 rr sinq f ËrrË r r2.2.1. The curl of EConsider a charge distribution r (r). The electric field at a point P generated by this chargedistribution is equal toE( r ) 1Dˆr2 r ( r ')dt 'Ú4pe 0 ( Dr )where Dr r - r '. The curl of E is equal to— E( r ) Ê1Dˆr ˆ— Á2 r (r ')dt 'Ú4pe 0 Ë( Dr ) - 9 -
However, — rˆ / r 2 0 for every vector r and we thus conclude that— E( r ) 02.2.2. Applications of Gauss's lawAlthough Gauss's law is always true it is only a useful tool to calculate the electric field if thecharge distribution is symmetric:1. If the charge distribution has spherical symmetry, then Gauss's law can be used withconcentric spheres as Gaussian surfaces.2. If the charge distribution has cylindrical symmetry, then Gauss's law can be used withcoaxial cylinders as Gaussian surfaces.3. If the charge distribution has plane symmetry, then Gauss's law can be used with pill boxesas Gaussian surfaces.Example: Problem 2.12Use Gauss's law to find the electric field inside a uniformly charged sphere (charge density r )of radius R.The charge distribution has spherical symmetry and consequently the Gaussian surface usedto obtain the electric field will be a concentric sphere of radius r. The electric flux through thissurface is equal toFE ÚE da 4p r 2 E( r )SurfaceThe charge enclosed by this Gaussian surface is equal toQEnclosed 4 3pr r3Applying Gauss's law we obtain for the electric field:- 10 -
4 31 QEnclosed1 3 pr rr rE( r ) 22e0e03e 04p r4p rExample: Problem 2.14Find the electric field inside a sphere which carries a charge density proportional to thedistance from the origin: r k r, for some constant k.The charge distribution has spherical symmetry and we will therefore use a concentric sphereof radius r as a Gaussian surface. Since the electric field depends only on the distance r, it isconstant on the Gaussian surface. The electric flux through this surface is therefore equal toFE ÚE da 4p r 2 E( r )SurfaceThe charge enclosed by the Gaussian surface can be obtained by integrating the chargedistribution between r' 0 and r' r:QEnclosed ÚVolumerr( r ')dt Ú0 kr'( 4p r '2 )dr ' p kr 4Applying Gauss's law we obtain:QEnclosed p kr 4F E 4p r E( r ) e0e02orÊ p kr 4 ˆÁË e 10E( r ) kr 224p r4e 0Example: Problem 2.16A long coaxial cable carries a uniform (positive) volume charge density r on the innercylinder (radius a), and uniform surface charge density on the outer cylindrical shell (radius b).The surface charge is negative and of just the right magnitude so that the cable as a whole isneutral. Find the electric field in each of the three regions: (1) inside the inner cylinder (r a),(2) between the cylinders (a r b), (3) outside the cable (b r).- 11 -
The charge distribution has cylindrical symmetry and to apply Gauss's law we will use acylindrical Gaussian surface. Consider a cylinder of radius r and length L. The electric fieldgenerated by the cylindrical charge distribution will be radially directed. As a consequence,there will be no electric flux going through the end caps of the cylinder (since here E da ). Thetotal electric flux through the cylinder is equal toFE ÚE da 2p rLE( r )SurfaceThe enclosed charge must be calculated separately for each of the three regions:1. r a:QEnclosed p r 2 Lr2. a r b:QEnclosed p a 2 Lr3. b r:QEnclosed 0Applying Gauss's law we findE( r ) 1 QEnclosed2p rL e 0Substituting the calculated Qencl for the three regions we obtain1. r a:1 QEnclosed1 pr 2 Lr1E( r ) rr .2p rL e 02p rL e 02e 02. a r b:E( r ) 1 QEnclosed1 pa 2 Lr1 a2 r2p rL e 02p rL e 02e 0 r3. b rE( r ) 1 QEnclosed 02p rL e 0Example: Problem 2.18Two spheres, each of radius R and carrying uniform charge densities of r and -r ,respectively, are placed so that they partially overlap (see Figure 2.6). Call the vector from thenegative center to the positive center s . Show that the field in the region of overlap is constantand find its value.To calculate the total field generated by this charge distribution we use the principle ofsuperposition. The electric field generated by each sphere can be obtained using Gauss' law (see- 12 -
Problem 2.12). Consider an arbitrary point in the overlap region of the two spheres (see Figure2.7). The distance between this point and the center of the negatively charged sphere is r-. Thedistance between this point and the center of the positively charged sphere is r . Figure 2.7shows that the vector sum of s and r is equal to r- . Therefore,r - r- -sThe total electric field at this point in the overlap region is the vector sum of the field due to thepositively charged sphere and the field due to the negatively charged sphere:Etot r(r - r )3e 0 -s Figure 2.6. Problem 2.18.E EtotE- -qs Figure 2.7. Calculation of Etot.- 13 -
The minus sign in front of r- shows that the electric field generated by the negatively chargedsphere is directed opposite to r- . Using the relation between r and r- obtained from Figure 2.7we can rewrite Etot asEtot -rs3e 0which shows that the field in the overlap region is homogeneous and pointing in a directionopposite to s .2.3. The Electric PotentialThe requirement that the curl of the electric field is equal to zero limits the number of vectorfunctions that can describe the electric field. In addition, a theorem discussed in Chapter 1 statesthat any vector function whose curl is equal to zero is the gradient of a scalar function. Thescalar function whose gradient is the electric field is called the electric potential V and it isdefined asE -— VTaking the line integral of — V between point a and point b we obtainbb— V dl V( b ) - V ( a ) - Úa E dlaÚTaking a to be the reference point and defining the potential to be zero there, we obtain for V(b)bV( b ) - Úa E dlThe choice of the reference point a of the potential is arbitrary. Changing the reference point ofthe potential amounts to adding a constant to the potential:babV'( b ) - Úa' E dl - Úa' E dl - Úa E dl K V( b )where K is a constant, independent of b, and equal toaK -Úa' E dlHowever, since the gradient of a constant is equal to zero- 14 -
E' -— V ' - — V EThus, the electric field generated by V' is equal to the electric field generated by V. The physicalbehavior of a system will depend only on the difference in electric potential and is thereforeindependent of the choice of the reference point. The most common choice of the referencepoint in electrostatic problems is infinity and the corresponding value of the potential is usuallytaken to be equal to zero:bV( b ) - Ú E dlThe unit of the electrical potential is the Volt (V, 1V 1 Nm/C).Example: Problem 2.20One of these is an impossible electrostatic field. Which one?a) E k ( xy)iˆ ( 2yz ) ˆj ( 3xz )kˆb)[]E k[( y )iˆ ( 2xy z ) ˆj ( 2yz )kˆ ]22Here, k is a constant with the appropriate units. For the possible one, find the potential, using theorigin as your reference point. Check your answer by computing — V .a) The curl of this vector function is equal to— EÊ ˆ Ê ˆ kÁ ( 3xz ) - ( 2yz ) ˆi kxy) - ( 3xz ) ˆj (Ë z Ë y z xÊ ˆ kÁ ( 2yz ) - ( xy) k -2yiˆ - 3zˆj - xkˆË x y()Since the curl of this vector function is not equal to zero, this vector function can not describe anelectric field.b) The curl of this vector function is equal to— EÊ ˆ Ê 2ˆ kÁ ( 2yz ) - ( 2xy z 2 ) ˆi ky ) - ( 2yz ) ˆj (Ë z Ë y z xÊ 2ˆ2kÁ ( 2xy z ) - ( y ) 0Ë x y- 15 -
Since the curl of this vector function is equal to zero it can describe an electric field. Tocalculate the electric potential V at an arbitrary point (x, y, z), using (0, 0, 0) as a reference point,we have to evaluate the line integral of E between (0, 0, 0) and (x, y, z). Since the line integralof E is path independent we are free to choose the most convenient integration path. I will usethe following integration path:0, 0, 0 Æ x , 0, 0 Æ x , y , 0 Æ x , y , zThe first segment of the integration path is along the x axis:dl dxiˆandE dl ky2 dx 0since y 0 along this path. Consequently, the line integral of E along this segment of theintegration path is equal to zero. The second segment of the path is parallel to the y axis:dl dyˆjandE dl k( 2xy z 2 )dy 2kxydysince z 0 along this path. The line integral of E along this segment of the integration path isequal to(x, y, 0)yE dl Ú0 2kxydy kxy2(x, 0, 0)ÚThe third segment of the integration path is parallel to the z axis:dl dzkˆandE dl 2k( yz )dzThe line integral of E along this segment of the integration path is equal to- 16 -
(x, y, z)zÚ(x, y, 0) E dl Ú0 2k( yz )dz kyz 2The electric potential at (x, y, z) is thus equal toV( x,y,z ) - Ú(x, 0, 0)(0, 0, 0)E dl - Ú(x, y, 0)(x, 0, 0)E dl - Ú(x, y, z)(x, y, 0)E dl 0 - kxy2 - kyz 2 -k( xy2 yz 2 )The answer can be verified by calculating the gradient of V:—V V ˆ V ˆ V ˆi j k -k y 2 ˆi ( 2xy z 2 ) ˆj ( 2yz )kˆ -E x y z()which is the opposite of the original electric field E .The advantage of using the electric potential V instead of the electric field is that V is a scalarfunction. The total electric potential generated by a charge distribution can be found using thesuperposition principle. This property follows immediately from the definition of V and the factthat the electric field satisfies the principle of superposition. SinceE E1 E2 E3 .it follows thatbbbbV -Ú E dl - Ú E1 dl - Ú E2 dl - Ú E3 dl - . V1 V2 V3 .This equation shows that the total potential at any point is the algebraic sum of the potentials atthat point due to all the source charges separately. This ordinary sum of scalars is in generaleasier to evaluate then a vector sum.Example: Problem 2.46Suppose the electric potential is given by the expressionV( r ) Ae -lrrfor all r (A and l are constants). Find the electric field E( r ) , the charge density r( r ) , and thetotal charge Q.- 17 -
The electric field E( r ) can be immediately obtained from the electric potential:E( r ) - — V ( r ) -Ê Ê e -lr ˆe -lre -lr ˆˆr A 2 rˆÁA Á lAËrr r Ë r The charge density r( r ) can be found using the electric field E( r ) and the following relation:— E( r ) r( r )e0This expression shows thatr( r ) e 0 [ — E ( r )]Substituting the expression for the electric field E( r ) we obtain for the charge density r( r ) :rˆ ˆ ÈÊr( r ) e 0 A Í — Á (1 l r )e -lr 2 Ër ÎÈ rˆ ˆÊrˆ e 0 A Í(1 l r )e -lr Á — 2 2 —((1 l r )e -lr ) Ër rÎ ÈÈl 2 e -lr l2e -lr 3 e 0 A Í 4p (1 l r )e -lrd 3 ( r ) eA4pdr()r 0 ÍÎr ÎThe total charge Q can be found by volume integration of r( r ) :Qtot r ( r )dtÚ Ú 0VolumeÈl 2 e -lr 32e 0 A Í 4pd ( r ) 4p r dr r Î 4pe 0 A ÈÍ Ú0 4pd 3 ( r )r 2 dr - Ú0 rl 2 e -lr dr Î -4pe 0 A Ú rl2e -lr dr0The integral can be solved easily:Ú 0-lrre dr -d -lrd Ê1ˆ 1edr 2Údl 0dl Ë l lThe total charge is thus equal to- 18 -
Qtot -4pe 0 AThe charge distribution r( r ) can be directly used to obtained from the electric potentialV( r )r( r ) e 0 [ — E ( r )] -e0 [ — — V ( r )] -e 0 — 2V( r )This equation can be rewritten as— 2V( r ) -r( r )e0and is known as Poisson's equation. In the regions where r( r ) 0 this equation reduces toLaplace's equation:— 2V( r ) 0The electric potential generated by a discrete charge distribution can be obtained using theprinciple of superposition:nVtot ( r ) ÂVi ( r )i 1where Vi ( r ) is the electric potential generated by the point charge qi . A point charge qi locatedat the origin will generate an electric potential Vi ( r ) equal toVi ( r ) -1 r qi1 qi2 dr' Ú4pe0 r '4pe 0 rIn general, point charge qi will be located at position ri and the electric potential generated bythis point charge at position r is equal toVi ( r ) 1qi4pe0 r - riThe total electric potential generated by the whole set of point charges is equal to1 n qiVtot ( r ) Â4pe0 i 1 r - ri- 19 -
To calculate the electric potential generated by a continuous charge distribution we have toreplace the summation over point charges with an integration over the continuous chargedistribution. For the three charge distributions we will be using in this course we obtain:1. line charge l : Vtot ( r ) 1ldl'Ú4pe0 Line r - r '2. surface charge s : Vtot ( r ) 1sda'Ú4pe0 Surface r - r '3. volume charge r : Vtot ( r ) 1rdt 'Ú4pe0 Volume r - r 'Example: Problem 2.25Using the general expression for V in terms of r find the potential at a distance z above thecenter of the charge distributions of Figure 2.8. In each case, compute E -— V . Suppose thatwe changed the right-hand charge in Figure 2.8a to -q. What is then the potential at P? Whatfield does this suggest? Compare your answer to Problem 2.2b, and explain carefully anydiscrepancy.a)PPb)zqPc)zzqld2LsFigure 2.8. Problem 2.35.a) The electric potential at P generated by the two point charges is equal to- 20 -R
V 14pe 0q1 2d z24 14pe 0q1 2d z24 12pe 0q1 2d z24The electric field generated by the two point charges can be obtained by taking the gradient ofthe electric potential:Ê Á 1E -— V - Á z Á 2pe 0Áˈ qqz1ˆ kˆ 3/ 2 k1 22pe 0 Ê 1 2ˆ2 2d z Ë4d z 4If we change the right-hand charge to -q then the total potential at P is equal to zero. However,this does not imply that the electric field at P is equal to zero. In our calculation we haveassumed right from the start that x 0 and y 0. Obviously, the potential at P will therefore notshow an x and y dependence. This however not necessarily indicates that the components of theelectric field along the x and y direction are zero. This can be demonstrated by calculating thegeneral expression for the electric potential of this charge distribution at an arbitrary point (x,y,z):V( x,y,z ) 14pe 0 q4pe 0q2 1 ˆÊx d y2 z 2Ë2 121 ˆÊd y 2 z 2x Ë2-14pe 0q4pe 0-q2 1 ˆÊx - d y2 z 2Ë2 121 ˆÊd y 2 z 2xË2The various components of the electric field can be obtained by taking the gradient of thisexpression:1 ˆÊË x 2 d 1 ˆÊË x - 2 d Ex( x,y,z ) - Vqq 3/ 2 2 x 4pe 0 Ê Ê4pe 0ˆ1 ˆ22Á Ë x d y z 2Ë Ey( x,y,z ) - Vqyqy 3/23/ 22 y 4pe 0 Ê Ê4pe 0 Ê Ê 1 ˆ 2ˆˆ1 ˆ2222Á Ë x d y z Á Ë x - d y z 22Ë Ë - 21 -ÊÊ 1 ˆ 2ˆ22Á Ë x - d y z 2Ë 3/ 2
Vqzqz 3/23/ 22 z 4pe 0 Ê Ê4pe 0 Ê Ê 1 ˆ 2ˆˆ1 ˆ2222Á Ë x d y z Á Ë x - d y z 22Ë Ë Ez( x,y,z ) -The components of the electric field at P (0, 0, z) can now be calculated easily:Ex( 0,0,z ) qd3/ 24pe 0 Ê 1 22ˆË4d z Ey( 0,0,z ) 0Ez( 0,0,z ) 0b) Consider a small segment of the rod, centered at position x and with length dx. The chargeon this segment is equal to l dx. The potential generated by this segment at P is equal todV 14pe 0l dxx2 z 2The total potential generated by the rod at P can be obtained by integrating dV between x - Land x L1-L 4pe0V ÚLl dx2x z2 lln4pe0[() (L2 z 2 L - lnL2 z 2 - L)]The z component of the electric field at P can be obtained from the potential V by calculating thez component of the gradient of V. We obtainEz( x,y,z ) -l ln4pe 0 z[() (L2 z 2 L - ln)]L2 z 2 - L zzÈ Í 2222l Íl2LL z L z 22224pe 0 Í L z LL z - L 4pe 0 z L2 z 2Í Î c) Consider a ring of radius r and width dr. The charge on this ring is equal to[2]dq s p ( r dr ) - p r 2 2p s rdr- 22 -
The electric potential dV at P generated by this ring is equal todV 14pe 0dq2r z 2s2e 0rdrr2 z2The total electric potential at P can be obtained by integrating dV between r 0 and r R:V s R rdrs Ú2e 0 0 r 2 z 2 2e 0[R2 z 2 - z]The z component of the electric field generated by this charge distribution can be obtained bytaking the gradient of V:Ez -s 2e 0 z[]R2 z 2 - z - s ÈzÍ 2-1 2e 0 ÍÎ R z 2 Example: Problem 2.5Find the electric field a distance z above the center of a circular loop of radius r, whichcarries a uniform line charge l.The total charge Q on the ring is equal toQ 2p RlThe total electric potential V at P is equal toV 14pe 02p Rl R2 z 2l2e 0RR2 z 2The z component of the electric field at P can be obtained by calculating the gradient of V:Ez -l 2e 0 zR2R z2 lRz22e 0 ( R z 2 )3/ 2This is the same answer we obtained in the beginning of this Chapter by taking the vector sum ofthe segments of the ring.We have seen so far that there are three fundamental quantities of electrostatics:- 23 -
1. The charge density r2. The electric field E3. The electric potential VIf one of these quantities is known, the others can be calculated:rKnown ØErE Er e 0— EVr -e0 — 2VV1rˆr dt4pe 0 Ú r 2V 1rdtÚ4pe 0 rV - Ú E dlE -— VIn general the charge density r and the electric field E do not have to be continuous. Considerfor example an infinitesimal thin charge sheet with surface charge s. The relation between theelectric field above and below the sheet can be obtained using Gauss's law. Consider arectangular box of height e and area A (see Figure 2.9). The electric flux through the surface ofthe box, in the limit e Æ 0, is equal toFE E da ( E above - E below )AÚSurfaceEaboveeAsEbelowFigure 2.9. Electric field near a charge sheet.- 24 -
where E ,above and E ,below are the perpendicular components of the electric field above andbelow the charge sheet. Using Gauss's law and the rectangular box shown in Figure 2.9 asintegration volume we obtainQencl sA E , above - E , below Ae0e0()This equation shows that the electric field perpendicular to the charge sheet is discontinuous atthe boundary. The difference between the perpendicular component of the electric field aboveand below the charge sheet is equal toE ,above - E , below se0The tangential component of the electric field is always continuous at any boundary. Thiscan be demonstrated by calculating the line integral of E around a rectangular loop of length Land height e (see Figure 2.10). The line integral of E , in the limit e Æ 0, is equal toÚ E dl Ú E ,above() dl Ú E ,below dl E ,above - E ,below LEaboveeEbelowLsFigure 2.10. Parallel field close to charge sheet.Since the line integral of E around any closed loop is zero we conclude that(E ,above)- E ,below L 0orE ,above E ,belowThese boundary conditions for E can be combined into a single formula:- 25 -
Eabove - Ebelow snˆe0where nˆ is a unit vector perpendicular to the surface and pointing towards the above region.The electric potential is continuous across any boundary. This is a direct results of thedefinition of V in terms of the line integral of E :belowVabove - Vbelow Úabove E dlIf the path shrinks the line integral will approach zero, independent of whether E is continuousor discontinuous. ThusVabove VbelowExample: Problem 2.30a) Check that the results of examples 4 and 5 of Griffiths are consistent with the boundaryconditions for E .b) Use Gauss's law to find the field inside and outside a long hollow cylindrical tube whichcarries a uniform surface charge s. Check that your results are consistent with the boundaryconditions for E .c) Check that the result of example 7 of Griffiths is consistent with the boundary conditions forV.a) Example 4 (Griffiths): The electric field generated by an infinite plane carrying a uniformsurface charge s is directed perpendicular to the sheet and has a magnitude equal toEabove s ˆk2e 0Ebelow -s ˆk2e 0Therefore,Eabove - Ebelow s ˆ s sheet ˆk ke0e0which is in agreement with the boundary conditions for E .- 26 -
Example 5 (Griffiths): The electric field generated by the two charge sheets is directedperpendicular to the sheets and has a magnitude equal toEI 0EII s ˆie0EIII 0The change in the strength of the electric field at the left sheet is equal toEII - EI s ˆ s left ˆi ie0e0The change in the strength of the electric field at the right sheet is equal toEIII - EII -s ˆ s right ˆi ie0e0These relations show agreement with the boundary conditions for E .b) Consider a Gaussian surface of length L and radius r. As a result of the symmetry of thesystem, the electric field will be directed radially. The electric flux through this Gaussiansurface is therefore
another charge Q (the test charge) we can use the principle of superposition. This principle states that the interaction between any two charges is completely unaffected by the presence of other charges. The force exerted on Q by q1, q2, and q3 (see Figure 2.1) is therefore equal to the vector sum of the force F 1 exerted by q1 on Q, the force F











