Transcription
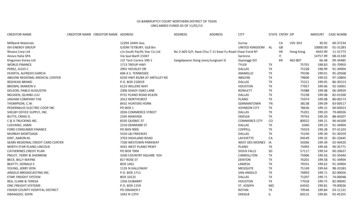
201-103-RE - Calculus 1WORKSHEET: LIMITS1. Use the graph of the function f (x) to answer each question.Use , or DN E where appropriate.(a)f (0) (b)f (2) (c)f (3) (d)(e)(f)(g)(h)lim f (x) x 0 lim f (x) x 0lim f (x) x 3 lim f (x) x 3lim f (x) x 2. Use the graph of the function f (x) to answer each question.Use , or DN E where appropriate.(a)f (0) (b)f (2) (c)f (3) (d)(e)(f)(g)lim f (x) x 1lim f (x) x 0lim f (x) x 2 lim f (x) x
3. Evaluate each limit using algebraic techniques.Use , or DN E where appropriate.(a)(b)limx2 25x2 4x 5(q)limx2 25x2 4x 5(r)x 0x 52(c)(d)(e)7x 4x 33x2 4x 1limx 1x4 5x3 6x2lim 2x 2 x (x 1) 4(x 1)lim x 1 x 3 (f)limx 3 (g)(h)(i)(j)(k)(l)(m)(n)(o)(p)(s)limx 33x(u)x 1 2x2 9(v)x2 7 3x 3(w)x2 2x 8x 2x2 5 (x 1) 2 1/32y 2y 4limy 56y 3plim 4 2 cos(x) 5lim x 011 lim 3 x 3 xx 0x12x 8 2lim x 12 xx 6x 6 lim x2 2 x2 1x limx lim 6x 7lim x 1(t)x 2 (x)(y)(z)(A)(B)(C)(D)x2x 143 3xlimx 3x3 x2 2x2 x 2x3 1x 5x 2x2 1 x5 1lim cosx x6 x5 100limlimx 22x 4x2limx 1 x23x 2x 1x2 25x 1 x2 4x 5 x2 5 2limx 3x 3lim2x sin(x)x 0x412lim exx 1 x 1limlim 2x2 3xx (E) x4 10x 4x3 xrx 3lim 3x 5 xlim(F) x 2 2 xlimx 0xexlim x 0 1 ln(x) lim x2 1 2xx 3x 1lim x 1x 1
4. Find the following limits involving absolute values.x2 1(a) limx 1 x 1 x2 x 3 (c) lim x 3x 31(b) lim x2x 2 x 2 5. Find the value of the parameter k to make the following limit exist and be finite.What is then the value of the limit?x2 kx 20limx 5x 56. Answer the following questions for the piecewise defined function f (x) described onthe right hand side.(a)f (1) (b)lim f (x) (c)x 0(sin(πx)f (x) lim f (x) 2x2for x 1,for x 1.x 17. Answer the following questions for the piecewise defined function f (t) described onthe right hand side.(a)f ( 3/2) (b)f (2) (c)f (3/2) (d)lim f (t) (e)t 2lim f (t) t 1 (f)lim f (t) (g)lim f (t) t 2t 0 t2 t 6f (t) t2 t 3t 2for t 2for 1 t 2for t 2
ANSWERS:(b) 0(c) 3(d) (b) DNE(c) 0(d) DNE1. (a) DNE2. (a) 0(e) DNE(f) 2(f) (e) 0(g) DNE(h) 1(g) 13.(a) 5(b)(l)53136(w) (m) 0(x) DNE(c) 5(n) DNE(d) 1(o) DNE(e) 1(p) 0(f)(g)12416(B) (s)(j) DNE(u) 1 29(C) 32(t) 04. (a) DNE(A) (r) 143(k)(z) (q) (h) 18(i)(y) DNE(D) 0(E) (F)(v) DNE(b) (c) 95. k 1, limit is then equal to 96. (a) DNE(b) 0(c) DNE7. (a) DNE(b) 4(c) 108. (a) 0(b) 0(c)53(d) DNE 12(e)52(f) 4(g) DNE23
NamePre-Calculus Rational functions worksheetFor each of the rational functions find: a. domainasymptotes e. y-intercept f. x-interceptsb. holesc. vertical asymptotesd. horizontal1. f x x2 x 2x2 x 62. f x 2 x2x2 13. f x 3x 24. f x 2x 1x5. f x x 2 x 12x2 96. f x x2 4x 3
7. f x x2 xx 18. f x 9. f x x 12x 3x 210. f x x2 x 2x 1x2 9x2 2 x 3
201-103-RE - Calculus 1WORKSHEET: CONTINUITY1. For each graph, determine where the function is discontinuous. Justify for eachpoint by: (i) saying which condition fails in the definition of continuity, and (ii) bymentioning which type of discontinuity it is.(a)(b)2. For each function, determine the interval(s) of continuity.(a) f (x) x2 ex3x 1(b) f (x) 22x 3x 2 45 x12 (d)* f (x) 4 x2x2 x 12(c) f (x) 3. For each piecewise defined function, determine where f (x) is continuous (or where itis discontinuous). Justify your answer in detail. 2x for x 0 x23 x2 3xfor x 1x2 3x for 0 x 2(a) f (x) (b) f (x) log10 (x) x for x 1 x2 8for x 2x4. Find all the value(s) of the parameter c (if possible), to make the given functioncontinuous everywhere.(c · 3x x2 2c for x 0(a) f (x) 2x5 c(x 1) 16 for x 0
(2(cx)3 x 1for x 12cx (x 1)2for x 1(b)f (x) (c) 3x c x2 cf (x) 3for x 1for 1 x 2for x 25.* Consider the function f (x) bxc, the greatest integer function (also called the floorfunction or the step function). Where is this function discontinuous?6.* Find an example of a function such that the limit exists at every x, but that hasan infinite number of discontinuities. (You can describe the function and/or write aformula down and/or draw a graph.)PARTIAL ANSWERS:1.2.3.4.5.6.(a) x 0, 3 (b) x 2, 0, 1(a) R (b) R\{ 1/2, 2} (c) ( , 5] (d) ( 3, 2) ( 2, 2) (2, 4)(a) discontinuous only at x 1 (b) discontinuous only at x 2(a) c 8 (b) c 1, 0, 1 (c) no solution possiblediscontinuous at every integer, x . . . , 3, 2, 1, 0, 1, 2, 3, . . .many answers are possible, show me your solution!
201-103-RE - Calculus 1WORKSHEET: DEFINITION OF THE DERIVATIVE1. For each function given below, calculate the derivative at a point f 0 (a)using the limit definition.(a) f (x) 2x2 3x (b) f (x) 2x 11(c) f (x) x 2f 0 (0) ?f 0 (4) ?f 0 (3) ?2. For each function f (x) given below, find the general derivative f 0 (x)as a new function by using the limit definition.(a) f (x) x 4(b) f (x) x3x(c) f (x) x 11(d) f (x) xf 0 (x) ?f 0 (x) ?f 0 (x) ?f 0 (x) ?3. For each function f (x) given below, find the equation of the tangent lineat the indicated point.(a) f (x) x x2at (2, 2)(b) f (x) 1 3x21(c) f (x) 2x (d) f (x) x xat (0, 1)at x 1at x 1ANSWERS:1. (a) f 0 (0) 3 (b) f 0 (4) 1/3 (c) f 0 (3) 112. (a) f 0 (x) 2 x 4(b) f 0 (x) 3x2 (c) f 0 (x) 3. (a) y 3x 4(b) y 1(c) y 12 x 11(x 1)2(d) f 0 (x) (d) y 32 x 12 12x3/2
Derivative Practice WorksheetSolve the derivatives for using basic differentiation.1. y 32. g x x 2 43. h t 2t 2 3t 64. s t t 3 2t 45.f x x 2 12 x3x46. y 5 x431727. g x 3x 5 xx8.f x x 2 12 x9. y 5 3 x 210. g x 1 3x 2x11. h x 13x312. y xx13. f x x3 3x 2 x 4Name:
14. y 3x 22x 315. f x 3 2 x x2x2 116. g x x 2 2 x 1 x3 1 17. y 3x 2 2 x 2 5x 18. f x 5x 2x2 1919. y x 420. f x 21. y x 1x4x922. y 4 x 2 3x 3x3 2 23. y 2 x2 x3x 224. y x2 2 x 13x
Worksheet # 12: Higher Derivatives and Trigonometric Functions1. Calculate the indicated derivative:(a) f (4) (1),f (x) x4(b) g (3) (5),g(x) 2x2 x 4(c) h(3) (t),h(t) 4et t3 s(w) wew(d) s(2) (w),2. Calculate the first three derivatives of f (x) xex and use these to guess a general formula for f (n) (x),the n-th derivative of f .3. Let f (t) t 2 cos(t).(a) Find all values of t where the tangent line to f at the point (t, f (t)) is horizontal.(b) What are the largest and smallest values for the slope of a tangent line to the graph of f ?4. Differentiate each of the following functions:(a) f (t) cos(t)1(b) g(u) cos(u)(c) r(θ) θ3 sin(θ)(d) s(t) tan(t) csc(t)(e) h(x) sin(x) csc(x)(f) f (x) x2 sin(x)(g) g(x) sec(x) cot(x)5. Calculate the first five derivatives of f (x) sin(x). Then determine f (8) and f (37)6. Calculate the first 5 derivatives of f (x) 1/x. Can you guess a formula for the nth derivative, f (n) ?7. A particle’s distance from the origin (in meters) along the x-axis is modeled by p(t) 2 sin(t) cos(t),where t is measured in seconds.(a) Determine the particle’s speed (speed is defined as the absolute value of velocity) at π seconds.(b) Is the particle moving towards or away from the origin at π seconds? Explain.3π(c) Now, find the velocity of the particle at time t . Is the particle moving toward the origin or2away from the origin?(d) Is the particle speeding up atπ2seconds?8. Find an equation of the tangent line at the point specified:(a) y x3 cos(x),x 0(b) y csc(x) cot(x),x (c) y eθ sec(θ),θ π4π49. Comprehension check for derivatives of trigonometric functions:(a) True or False: If f 0 (θ) sin(θ), then f (θ) cos(θ).2(b) True or False: If θ is one of the non-right angles in a right triangle and sin(θ) , then the3hypotenuse of the triangle must have length 3.
Math Excel Supplemental Problems #121. Use the Product Rule twice to find a formula for (f g)00 in terms of f and g as well as their first and secondderivatives.2. Calculate the first and second derivatives of the following functions:(a) f (x) x sin x(b) f (x) (c) f (x) excos xcsc xx(d) f (x) tan x3. Calculate the first five derivatives of f (x) cos x, then determine f (8) and f (37)
CalculusChain Rule Practice s 92B0T1F34 QKZuut4a8 RSCohfgtzwbaorFeA CLtLhCQ.P L YA0lhlA 2rJiJgHhBt9sq Pr9eGszecrqvRevde.2Differentiate each function with respect to x. 2) y x 1) y ( x ) 3) f ( x) ( x ) x 5) y ( x )( x ) C N2S0c1h3j dKJuntvaP zS7oIfktdweaNrdeR nLQLJCN.y a ZA0luln MrlitgQhftfsS prbe4sHehrevPe2dB.k j WM1a0deet 4wtiCtlh2 CInnMf8iKnliVtZer qCnaKlscDuKlGursL.e4) y x ( x ) x 6) f ( x) x (-1-) Worksheet by Kuta Software LLC
(7) f ( x) x x ) 8)9) y sec x x x 10) f ( x) ( x )csc x 11) f ( x) cos x x 13) f ( x) f ( x) x tan x R e2g0C163m zKJu8tDaI fSBoMfytJwsaOrMeX XLlLlC2.A k tAelQl6 qrZiWgWhttase 7rGezsWebrYvgehdd.m Q 8M9aHdVe5 cwViJtyhL wIqnffyi0nhistveu UCia2lrcvuPluuwsZ.N12) f ( x) sin x 14) y cot-2- x Worksheet by Kuta Software LLC
Answers to Chain Rule Practice1) dy ( x ) x dx x ( x ) dy 2) ( x ) x dx x ( x ) ( x ) x x 3) f ' ( x) ( x ) ( x ) ( x ) x dy4) ( x ) ( x ) ( x ) x dx ( x ) ( x x ) ( x ) dy ( x ) ( x ) x ( x ) dx ( x x ) ( x ) ( x ) x ( x ) x x 6) f ' ( x) x ( x ) 5)( ) x ( x ) ( x x )( x ) ( x ) x ( x ) x x 7) f ' ( x) x ( x ) x( x x ) ( ) ( x ) ( x ) 8) f ' ( x) ( x ) ( x ) x ( x ) 9)( x ) dy sec x tan x x dx x sec x tan x x x ( x ) ( x )10) f ' ( x) ( x ) csc x cot x x csc x x x csc x ( x cot x xcot x ) T M2G0j1f3F XKTuvt3an iSpoQf2t9wOaRrtem HLNL4CF.y c CA9l5lW urYimghh1tTsy mr6eOs5eVr3vkejdW.I d 2MvatdteI Nw5intkhZ oI5n1fFivnNiVtvev 4C3atlycRu2lWu7s1.2-3-Worksheet by Kuta Software LLC
11) f ' ( x) cos x ( x ) x ( x ) sin x x x( x sin x sin x xcos x ) ( x )12) f ' ( x) cos x x x cos x tan x x ( x ) sec x x ()13) f ' x tan x x ( tan x x sec x x sec x ) tan x dy14) csc ( x ) ( x ) x dx x csc ( x )( x ) f g2D0G1K39 VKiumtVaq RSBobfbtnwCaUrKeH lLELRCh.3 b FAbldlV zr9i9gDhJtZs2 HrEeKsjeMrtvmeXdL.f f FMdardvek mw9ietEhV RIDnyf9iWnfi0tTeT rC1aLlqcPuVlquIsO.K-4-Worksheet by Kuta Software LLC
Implicit differentiation worksheet for Calculus 1Determine dy/dx for each of the following.(1)(2)(3)(4)(5)(6)(7)(8)y x2 xyx2 y y 3x1/4 y 1/4 2x1/3 y 1/3 7 x y 25x2 y 2 1.1x3 y 3 5x sin(y) y 1(9)(10)(11)(12)(13)(14)(15)(16) y x x y 16x2 xy y 3 xy 2x2 y 2 7x2/3 y 2/3 a2/3 (a is a constant)xa y 2 xb y xc 0 (a, b, c constants)sin(xy) 2x 5x ln(y) y 3 ln(x)ecos(y) x3 sin(y)Determine d2 y/dx2 for each of the following.(17) 1 xy x y 2(18) x y (x y)2(19) x2/3 y 2/3 8(20) sin(x) 4 cos(y) y5-80-5For the curve x2 y 2 xy 3x 9 0 (above),(21)(22)(23)(24)Determine dy/dx.Where do the horizontal tangent lines occur?Where do the vertical tangent lines occur (dy/dx )?Determine d2 y/dx2 .8
42-4-2024-2-4For the curve x2 xy y 2 5 (above),(25) Determine dy/dx.(26) Where do the horizontal tangent lines occur?(27) Where do the vertical tangent lines occur (dy/dx )?(28) Determine d2 y/dx2 .Consider the equation(29)(30)(31)(32)(cos x)y 2 (3 sin x 1)y (7x 2) 0Check that x 0, y 2 satisfies this equation.Find dy/dx at the point (0, 2) using implicit differentiation.Use the quadratic formula to solve for y in terms of x. (Should you use “ ” or “ ”? Why?)Would you like to find dy/dx using that formula for y? (Me neither.)Find f 0 (x) in terms of g(x) and g 0 (x), where g(x) 0 for all x. (Hint: if a is a constant then g(a) isconstant.)f (x) g(x)3f (x) g(x)(x a)f (x) g(a)(x a)f (x) g(x g(x))g(x)(37) f (x) x a(33)(34)(35)(36)1g(x)f (x) g(xg(a))pf (x) pg(x)2f (x) g(x2 )f (2x 3) g(x2 )(38) f (x) (39)(40)(41)(42)
Kuta Software - Infinite CalculusNameLogarithmic DifferentiationDate PeriodUse logarithmic differentiation to differentiate each function with respect to x.1) y 2 x2x2) y 5 x3) y 3 x3x4) y 4 x5) y (3 x 4 4) 37)5x3 1(3 x 4 2) 5y (3 x 3 4) 2 6 h2v0a102Q 1KwuktxaM ASFoyfPtMwgaXrGev 1L4LwCC.3 J SAhlSla 1rWihgahvtQs8 IrxefsEe0rfvveddg.i w uMqaDdcer EwhiCtxhz MI8ntfGiwnIictIec QCtaslYcSuNlLufsq.45xx46) y ( x 5 5) 28) y -1-2x2 33 x 2 1 (3 x 4 1) 3Worksheet by Kuta Software LLC
9) y 2x3 310) y (2 x 2 5) 3x2 2( x 4 3) 3Use logarithmic differentiation to differentiate each function with respect to x. You do not need tosimplify or substitute for y.11)4(5 x 4) 4y (3 x 2 5) 5 (5 x 4 3) 313) y (5 x 5 2) 2 (3 x 3 1) 3 (3 x 1) 453215) y (3 x 3 4) (3 x 1) (5 x 3 2) ( x 3)16) y 212) y ( x 2) (2 x 5) (5 x 1)14)3( x 2 3) 4y (5 x 5 2) 5 (3 x 2 5) 24(4 x 2 5) 2(2 x 3) 4 (5 x 4 2) 5 (3 x 2 4) 3 Z X2w031924 dK4uSt9aG VSto5fGtLwraErbef XLELFCB.u 3 NAHlLla mrCi9gFhNtZs5 grOeksIeNrBvveudE.u G BMVaqdbee owNiGtRhM 9I8nKfjiRnUiKt4e4 MCua2lbccuLl2uksz.3-2-Worksheet by Kuta Software LLC
Kuta Software - Infinite CalculusNameLogarithmic DifferentiationDate PeriodUse logarithmic differentiation to differentiate each function with respect to x.1) y 2 x2x2) y 5 xdy y(2 ln x 2)dx2x 4 x (ln x 1)3) y 3 xdy y(5 ln x 5)dx5x 25 x (ln x 1)3x4) y 4 xdy y(3 ln x 3)dx3x 9 x (ln x 1)5) y (3 x 4 4) 37)6) y ( x 5 5) 25x3 1(8) y )3 x 2 1 (3 x 4 1) 33x36 x 3dy y 2 4dx3x 13x 1243 x(3 x 1) (39 x 4 1 12 x 2 ) 3x2 1(60 x 318 x 2dy y 4 dx3x 23x3 446 x 2 (3 x 4 2) (21 x 4 40 x 6) (3 x 3 4) 3) Q x2S001D2N 8KLuutaa6 JSWoffjtOw9aUr9el 3LgLKCs.H v lAblBlv crpiBgNhztssh jr9ewsxeFrivee5d6.K k ZMWa7dCeg wwEiWt6hn zIcnmfwiqn8igtFeb qCaaJlEcSuClEuosb.u2x2 310 x 42xdy y 5 2dxx 52x 352 x( x 5)(11 x 5 15 x 3 5) 2x2 3)(3 x 4 2) 5y (3 x 3 4) 2(x4dy y(4 x 3 ln x x 3 )dxx4 3(4 ln x 1) 4x36 x 315 x 2dy y 4 dx3x 410 x 3 23 x 2 (3 x 4 4) 2 (135 x 4 24 x 20) 2 5x3 1(5x-1-)Worksheet by Kuta Software LLC
2x3 39) y 10) y (2 x 2 5) 3( x 4 3) 33x212 x 3dy y 3 4dx2x 3x 3243 x ( 7 x 3 12 x) ( x 4 3) 4 2 x 3 3(x2 2()12 xxdy y 2 2dx2x 5x 22x(2 x 2 5) (14 x 2 29) x2 2)Use logarithmic differentiation to differentiate each function with respect to x. You do not need tosimplify or substitute for y.11)4(5 x 4) 4y (3 x 2 5) 5 (5 x 4 3) 33(2030 x60 xdy y 2dx5x 43x 55x4 3((250 x27 x12dy y 5 3 dx5x 23x 1 3x 153)14))2( x 2 3) 4y (5 x 5 2) 5 (3 x 2 5) 2(445 x 2930 x 24dy y 3 3 dx3x 43x 1 5x 2 x 3)16) y )8x125 x 412 xdy y 2 5dxx 35x 23x2 515) y (3 x 3 4) (3 x 1) (5 x 3 2) ( x 3)(34415dy y dxx 2 2x 5 5x 113) y (5 x 5 2) 2 (3 x 3 1) 3 (3 x 1) 44212) y ( x 2) (2 x 5) (5 x 1))(4 x 2 5) 2(2 x 3) 4 (5 x 4 2) 5 (3 x 2 4) 316 x8100 x 318 xdy y 2 4dx4x 52x 35x 23x2 4()Create your own worksheets like this one with Infinite Calculus. Free trial available at KutaSoftware.com U W240i1l2k LKGuat4aF 7SmojfDtvwsaFrCeh wLILVCS.X Y uAQlRl8 qrIiDgghAtusW Rr8etseexrMvzesd9.S Z BMHa7dAea ow5iItDhj mIQn4frinnDintUeh vCyarl4cAuXluu7sn.G-2-Worksheet by Kuta Software LLC
6.4 Exponential Growth and DecayCalculus6.4 EXPONENTIAL GROWTH AND DECAYIn many applications, the rate of change of a variable y is proportional to the value of y. If y is a function of time t,we can express this statement asExample: Find the solution to this differential equation given the initial condition that y y0 when t 0.(This is the derivation of an exponential function see notecards)Exponential Growth and Decay ModelIf y changes at a rate proportional to the amount present ( dy ky ) and y y0 when t 0, thendty y0 ektwhere k is the proportional constant.Exponential growth occurs when k 0 , and exponential decay occurs when k 0 .Example: The rate of change of y is proportional to y. When t 0, y 2. When t 2, y 4. What is the value of ywhen t 3?Example: [1985 AP Calculus BC #33] IfA) 12 ln 2B) 14dydt 2 y and if y 1 when t 0, what is the value of t for which y 12 ?C)12ln 2146D)22E) ln 2
6.4 Exponential Growth and DecayCalculusExample: Radioactive Decay: The rate at which a radioactive element decays (as measuredby the number of nuclei that change per unit of time) is approximately proportional to theamount of nuclei present. Suppose that 10 grams of the plutonium isotope Pu-239 wasreleased in the Chernobyl nuclear accident. How long will it take for the 10 grams to decay to1 gram? [Pu-239 has a half life of 24,360 years]Example: Newton’s Law of Cooling: Newton’s Law of Cooling states that therate of change in the temperature of an object is proportional to the differencebetween the object’s temperature and the temperature in the surrounding medium.A detective finds a murder victim at 9 am. The temperature of the body ismeasured at 90.3 F. One hour later, the temperature of the body is 89.0 F. Thetemperature of the room has been maintained at a constant 68 F.(a) Assuming the temperature, T, of the body obeys Newton’s Law ofCooling, write a differential equation for T.(b) Solve the differential equation to estimate the time the murder occurred.147
6.4 Exponential Growth and DecayCalculusExample: [1988 AP Calculus BC #43] Bacteria in a certain culture increase at rate proportional to the numberpresent. If the number of bacteria doubles in three hours, in how many hours will the number of bacteria triple?A)3ln 3ln 2B)2 ln 3ln 2C)ln 3ln 2 27 D) ln 2 9 E) ln 2 Example: [AP Calculus 1993 AB #42] A puppy weighs 2.0 pounds at birth and 3.5 pounds two months later. If theweight of the puppy during its first 6 months is increasing at a rate proportional to its weight, then how much willthe puppy weigh when it is 3 months old?A) 4.2 poundsB) 4.6 poundsC) 4.8 poundsD) 5.6 poundsE) 6.5 poundsExample: [1993 AP Calculus BC #38] During a certain epidemic, the number of people that are infected at any timeincreases at rate proportional to the number of people that are infected at that time. If 1,000 people are infectedwhen the epidemic is first discovered, and 1,200 are infected 7 days later, how many people are infected 12 daysafter the epidemic is first discovered?A) 343B) 1,343C) 1,367D) 1,400Example: [1998 AP Calculus AB #84] Population y grows according to the equationE) 2,057dydt ky , where k is a constantand t is measured in years. If the population doubles every 10 years, then the value of k isA) 0.069B) 0.200C) 0.301Notecards from Section 6.4: Derivation of an exponential function148D) 3.322E) 5.000
Kuta Software - Infinite CalculusNameRelated RatesDate PeriodSolve each related rate problem.1) Water leaking onto a floor forms a circular pool. The radius of the pool increases at a rate of 4cm/min. How fast is the area of the pool increasing when the radius is 5 cm?2) Oil spilling from a ruptured tanker spreads in a circle on the surface of the ocean. The area ofthe spill increases at a rate of 9π m²/min. How fast is the radius of the spill increasing when theradius is 10 m?3) A conical paper cup is 10 cm tall with a radius of 10 cm. The cup is being filled with water sothat the water level rises at a rate of 2 cm/sec. At what rate is water being poured into the cupwhen the water level is 8 cm? f j2I0n1u3h MKRuat8aD mSNowfVtzwjaKrQe6 tLsLzCt.F H fADlPlu 9rwiEgHhntzsL iriems3eorYv4eNdU.4 9 XMGasdFew Tw8iztchh 9IYnXfri7nMiVtveS VCxailic6uHlYuisJ.4-1-Worksheet by Kuta Software LLC
4) A spherical balloon is inflated so that its radius (r) increases at a rate of2cm/sec. How fast isrthe volume of the balloon increasing when the radius is 4 cm?5) A 7 ft tall person is walking away from a 20 ft tall lamppost at a rate of 5 ft/sec. Assume thescenario can be modeled with right triangles. At what rate is the length of the person's shadowchanging when the person is 16 ft from the lamppost?6) An observer stands 700 ft away from a launch pad to observe a rocket launch. The rocketblasts off and maintains a velocity of 900 ft/sec. Assume the scenario can be modeled as a righttriangle. How fast is the observer to rocket distance changing when the rocket is 2400 ft fromthe ground? q M2y0b1z39 fKTuqt6aI lSLoEf1tow6a9rieQ HLuLOCS.h X dAgl4lJ drIi2g2hgtFsW XrkeeshexrPvXe9dS.3 1 pMua3dyem vwQi2tBhJ TIRn0fpi7nXictOej BCraulDcouvlkuPsp.B-2-Worksheet by Kuta Software LLC
Kuta Software - Infinite CalculusNameRelated RatesDate PeriodSolve each related rate problem.1) Water leaking onto a floor forms a circular pool. The radius of the pool increases at a rate of 4cm/min. How fast is the area of the pool increasing when the radius is 5 cm?A area of circle r radiusEquation: A π r2t timedrdAGiven rate: 4 Find:dtdtr 5dAdtdr 2πr 40π cm²/mindtr 52) Oil spilling from a ruptured tanker spreads in a circle on the surface of the ocean. The area ofthe spill increases at a rate of 9π m²/min. How fast is the radius of the spill increasing when theradius is 10 m?A area of circle r radiusEquation: A π r 2t timedAGiven rate: 9πdtFind:drdtr 10drdt 1 dA9 m/min2πr dt 20r 103) A conical paper cup is 10 cm tall with a radius of 10 cm. The cup is being filled with water sothat the water level rises at a rate of 2 cm/sec. At what rate is water being poured into the cupwhen the water level is 8 cm?V volume of material in cone h height t timeπh 3dhdVEquation: V Given rate: 2 Find:3dtdth 8dVdtdh πh 2 128π cm³/secdth 8 2 y2q0d1G34 cKKultUaW ySWokfxtKwzamrHeJ eL8L4Co.0 I pA3lclb prniogMhUtOs4 lrRees2eFrgvjeKdb.j S zMBa8dgen kw9iftehQ AIPnJfRignUiQtQes PCoapl3cOu6l3uksS.4-1-Worksheet by Kuta Software LLC
4) A spherical balloon is inflated so that its radius (r) increases at a rate of2cm/sec. How fast isrthe volume of the balloon increasing when the radius is 4 cm?V volume of sphere r radius t time4 3dr 2dVEquation: V π r Given rate: Find:dt rdt3r 4dVdt 4π r 2 dr 32π cm³/secdtr 45) A 7 ft tall person is walking away from a 20 ft tall lamppost at a rate of 5 ft/sec. Assume thescenario can be modeled with right triangles. At what rate is the length of the person's shadowchanging when the person is 16 ft from the lamppost?x distance from person to lamppost y length of shadow t timex y ydxdyEquation: Given rate: 5 Find:207dtdtx 167 dx 35 ft/sec13 dt 13dydtx 166) An observer stands 700 ft away from a launch pad to observe a rocket launch. The rocketblasts off and maintains a velocity of 900 ft/sec. Assume the scenario can be modeled as a righttriangle. How fast is the observer to rocket distance changing when the rocket is 2400 ft fromthe ground?a altitute of rocketz distance from observer to rocket t timedadzEquation: a 2 490000 z 2 Given rate: 900 Find:dtdta 2400a da 864 ft/secz dtdzdta 2400Create your own worksheets like this one with Infinite Calculus. Free trial available at KutaSoftware.com f 72F0g1f3z XK5untPaA xS7okfytNwOa7rKeh uLhLiC8.i M GAIlelf erfiHgThXtGs1 5rLe3sle4r9vQeidj.m 6 yM8a6dLel fw6ibt6hv DIAnbfgiInTiktveN lCmaplycjuPlOuisH.w-2-Worksheet by Kuta Software LLC
CalculusName4.6 Related RatesDate Period 8 b2305193o lK0uCt5ai FSHopfitcwkadr9ee MLBL1Cv.0 h cA5lrlx 8rzi8gThztZs9 2rJejsqeprTvCeVdy.wSolve each related rate problem.1) A spherical balloon is deflated so that its radius decreases at a rate of 4 cm/sec. At what rate isthe volume of the balloon changing when the radius is 3 cm?256πcm³/sec. At what rate is the radius of the3balloon changing when the radius is 8 cm?2) A spherical balloon is deflated at a rate of3) Water leaking onto a floor forms a circular pool. The radius of the pool increases at a rate of 9cm/min. How fast is the area of the pool increasing when the radius is 12 cm?4) A 7 ft tall person is walking towards a 17 ft tall lamppost at a rate of 4 ft/sec. Assume thescenario can be modeled with right triangles. At what rate is the length of the person's shadowchanging when the person is 12 ft from the lamppost?5) A conical paper cup is 30 cm tall with a radius of 10 cm. The cup is being filled with water at a2πrate ofcm³/sec. How fast is the water level rising when the water level is 2 cm?36) A 13 ft ladder is leaning against a wall and sliding towards the floor. The top of the ladder issliding down the wall at a rate of 7 ft/sec. How fast is the base of the ladder sliding away fromthe wall when the base of the ladder is 12 ft from the wall?7) Oil spilling from a ruptured tanker spreads in a circle on the surface of the ocean. The radius ofthe spill increases at a rate of 2 m/min. How fast is the area of the spill increasing when theradius is 13 m?8) A hypothetical cube shrinks so that the length of its sides are decreasing at a rate of 2 m/min.At what rate is the volume of the cube changing when the sides are 2 m each?9) A conical paper cup is 10 cm tall with a radius of 10 cm. The bottom of the cup is puncturedso that the water level goes down at a rate of 2 cm/sec. At what rate is the volume of water inthe cup changing when the water level is 9 cm?10) An observer stands 500 ft away from a launch pad to observe a rocket launch. The rocketblasts off and maintains a velocity of 700 ft/sec. Assume the scenario can be modeled as a righttriangle. How fast is the observer to rocket distance changing when the rocket is 1200 ft fromthe ground?11) A spherical snowball melts at a rate of 36π in³/sec. At what rate is the radius of the snowballchanging when the radius is 5 in? g 52P0l133e 5Ktu3tlaY tSWoBfRtcwSawrkeQ mLzLzCD.u 2 7AklGlf lrbiEgkhjtbsA 9rlewsSeIr2vPeVdW.L 2 7Mza5dWeI gwbiMtMhN bImnFf0iEnEistUet SCDallJcru1lsuTsG.k-1-Worksheet by Kuta Software LLC
12) A hypothetical cube grows at a rate of 8 m³/min. How fast are the sides of the cube increasingwhen the sides are 2 m each?13) A conical paper cup is 10 cm tall with a radius of 30 cm. The cup is being filled with water sothat the water level rises at a rate of 2 cm/sec. At what rate is water being poured into the cupwhen the water level is 9 cm?14) Water slowly evaporates from a circular shaped puddle. The radius of the puddle decreases ata rate of 8 in/hr. Assuming the puddle retains its circular shape, at what rate is the area of thepuddle changing when the radius is 3 in?15) A hypothetical square grows so that the length of its diagonals are increasing at a rate of 4m/min. How fast is the area of the square increasing when the diagonals are 14 m each?16) Water slowly evaporates from a circular shaped puddle. The area of the puddle decreases at arate of 16π in²/hr. Assuming the puddle retains its circular shape, at what rate is the radius ofthe puddle changing when the radius is 12 in?17) A hypothetical cube grows so that the length of its sides are increasing at a rate of 4 m/min.How fast is the volume of the cube increasing when the sides are 7 m each?18) A hypothetical square grows at a rate of 16 m²/min. How fast are the sides of the squareincreasing when the sides are 15 m each?19) A hypothetical cube shrinks at a rate of 8 m³/min. At what rate are the sides of the cubechanging when the sides are 3 m each?20) A spherical snowball melts so that its radius decreases at a rate of 4 in/sec. At what rate is thevolume of the snowball changing when the radius is 8 in?21) A perfect cube shaped ice cube melts so that the length of its sides are decreasing at a rate of 2mm/sec. Assume that the block retains its cube shape as it melts. At what rate is the volume ofthe ice cube changing when the sides are 2 mm each?22) A conical paper cup is 10 cm tall with a radius of 10 cm. The bottom of the cup is punctured9πcm³/sec. At what rate is the water level changingso that the water leaks out at a rate of4when the water level is 6 cm?23) A hypothetical square shrinks so that the length of its diagonals are changing at a rate of 8m/min. At what rate is the area of the square changing when the diagonals are 5 m each?24) A hypothetical square shrinks at a rate of 2 m²/min. At what rate are the diagonals of thesquare changing when the diagonals are 7 m each?25) Water leaking onto a floor forms a circular pool. The area of the pool increases at a rate of25π cm²/min. How fast is the radius of the pool increasing when the radius is 6 cm? d w2E0W1n3n xKPu8t5ae JSuo2fqtowza9rSeK pL4LiCV.P O 2A9lZl6 ir6idgHhOtDs7 br0eesWePrvvOeYdF.1 O WMaaidGeH PwTimtMh7 ZI4nWfniNnciOtIeI MCva7lJcJuuleuMs9.A-2-Worksheet by Kuta Software LLC
Answers to 4.6 Related Rates1) V volume of sphere r radius t time4 3drdVEquation: V π r Given rate: 4 Find:3dtdtr 3dVdtdr 4πr 2 144π cm³/secdtr 32) V volume of sphere r radius t time4 3256πdVEquation:
4. Find the following limits involving absolute values. (a) lim x!1 x2 1 jx 1j (b) lim x! 2 1 jx 2j x2 (c) lim x!3 x2jx 3j x 3 5. Find the value of the parameter kto make the following limit exist and be nite. What is then the value of the limit? lim x!5 x2 kx 20 x 5 6. Answer the foll