Transcription
B6P (x , y )1OqxyQATRIGONOMETRYThere is perhaps nothing which so occupies the middleposition of mathematics as Trigonometry.- J. F. HerbartEuler, like Newton, was the greatestmathematician of his generation. Hestudied all areas of mathematics andcontinued to work hard after he hadgone blind. Euler made discoveries inmany areas of mathematics, especiallyLeonhardEulerAryabhatta(AD(CE)1707(A.D (CE) 476- –1783)550)Calculus and Trigonometry. He was the first to proveseveral theorems in Geometry.Learning OutcomesÂÂ To understand the relationship among various trigonometric ratios.ÂÂ To recognize the values of trigonometric ratios and their reciprocals.ÂÂ To use the concept of complementary angles.ÂÂ To understand the usage of trigonometric tables.6.1 IntroductionTrigonometry (which comes from Greek words trigonon means triangle and metronmeans measure) is the branch of mathematics that studies the relationships involvinglengths of sides and measures of angles of triangles. It is a useful tool for engineers,scientists, and surveyors and is applied even in seismology and navigation.Observe the three given right angled triangles; in particular scrutinize theirmeasures.The corresponding angles shown in the three triangles are of the samesize. Draw your attention to the lengths of “opposite” sides (meaning the side oppositeto the given angle) and the “adjacent” sides (which is the side adjacent to the givenangle) of the triangle.2226 Trigonometry.indd 2229th Standard Mathematics23-02-2019 14:18:22
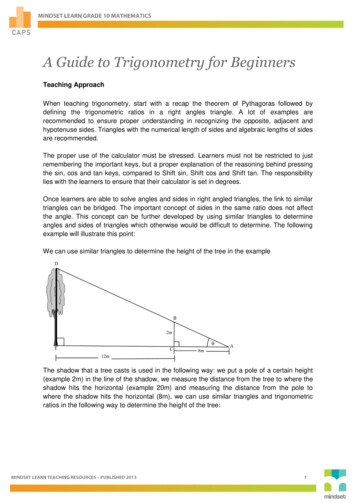
I3.5 units35 A 5 units7 unitsC14 unitsF35 35 B DEG10 unitsH20 unitsFig. 6.1 opposite side What can you say about the ratio adjacent side in each case? Every right angled triangle given here has the same ratio 0.7 ; based on this finding, now what could be theClength of the side marked ‘x’ in the Fig 6.2? Is it 15?remarkableratiosstunnedearly10.5 unitsSuchmathematicians and paved the way for the subject oftrigonometry.35 There are three basic ratios in trigonometry, eachABx unitsFig. 6.2of which is one side of a right-angled triangle dividedby another.sinecosinetangentShort formsincostanCRelatedmeasurementspohyte nuseqARelationshipsinθ Bopposite sidehypotenuseopposite side of angle θName of theangleCChyteponuseqAAadjacent side of angle θcosθ Badjacent sidehypotenuseqadjacent side of angle θtanθ Bopposite side of angle θThe three ratios are:opposite sideadjacent sideTrigonometry 2236 Trigonometry.indd 22323-02-2019 14:18:24
For the measures in the figure, compute sine, cosine and tangentratios of the angle q .35 unitsRSolutionIn the given right angledtriangle, note that for the given angleq , PR is the ‘opposite’ side and PQ isthe ‘adjacent’ side.sinθ opposite side PR 35 hypotenuseQR 37cosθ adjacent side PQ 12 hypotenuseQR 37tanθ opposite side PR 35 adjacent side PQ 1237 uP12 unitsExample 6.1n it sqFig. 6.3QIt is enough to leave the ratios as fractions. In case, if you want to simplify each rationeatly in a terminating decimal form, you may opt for it, but that is not obligatory.Note Since trigonometric ratios are defined in terms of ratios of sides, they are unitless numbers. Ratios like sinθ, cosθ, tanθ are not to be treated like (sin) (θ), (cos) (θ),(tan) (θ).Thinking CornerThe given triangles ABC,DEFand GHI have measuresI3-4-5, 6-8-10 and 12-16-20.Are they all right triangles?How do you know?The angles at the vertices B, E and Hare of equal size (each angle is equal to θ).With these available details, fill up thefollowing table and comment on the ratiosthat you get.2246 Trigonometry.indd 224F6 3D105AG20C124qB816qFig. 6.4EqH9th Standard Mathematics23-02-2019 14:18:27
In ABC3sin q 5cos q ?tanq 34In DEF6sin q ?10cos q ?In GHI12sin q ?20cos q ?tan q ?tan q ?Reciprocal ratiosWe defined three basic trigonometric ratios namely, sine, cosine and tangent. Thereciprocals of these ratios are also often useful during calculations. We define them as follows:Basic TrigonometricRatiosopposite sidesin θ hypotenusecos θ tan θ Its reciprocalcosecant θ adjacent sideopposite sideadjacent sidehypotenuseopposite sidecotangent θ cosec θ hypotenuseadjacent sidesecant θ hypotenuseShort formsec θ adjacent sidecot θ opposite sidehypotenuseopposite sidehypotenuseadjacent sideadjacent sideopposite sideFrom the above ratios we can observe easily the following relations:111cosec θ sec θ cot θ tanqsinqcos q111sinθ cos θ tan θ secqcosecqcot q(sin q ) (cosec q ) 1 . We usually write this as sin θ cosec θ 1.(cos q ) (sec q ) 1 . We usually write this as cos θ sec θ 1.(tan q ) (cot q ) 1. We usually write this as tan θ cot θ 1.Example 6.2Find the six trigonometric ratiosof the angle q using the given diagram.7SolutionBy Pythagoras theorem, (25)2 72 625 49 576 2425qAFig. 6.5Copposite side ofangle θAB BC 2 AC 2C7ABhypotenuse2524adjacent side of angle θFig. 6.6qBTrigonometry 2256 Trigonometry.indd 22523-02-2019 14:18:41
The six trignometric ratios aretanq secq opposite side 725cos q 724cosecq hypotenuse 25 adjacent side 24cotq hypotenuseopposite sideadjacent sideExample 6.3adjacent sidehypotenusehypotenuse 25 opposite side 7adjacent sideopposite sidean gCntacea djBFig. 6.7AC 13opposite side hypotenuse213 cos Ahypotenuse13 opposite side2sec A cot A If sec q Let BC 13 and AB 5hypotenuseBC 13 adjacent side AB 5By the Pythagoras theorem,2266 Trigonometry.indd 2269th2adjacent side hypotenuseopp2 si d eteo siaofng leΑ313hypotenuse13 adjacent side3adjacent sideopposite side 32C132 sin q 3 cos q, then show that354 sin q 9 cos qSolution:AC BC ABse332 22 9 4 132t e nuofsi d eAC AB 2 BC 2secq 247le A2tan A adjacent side 3By Pythagoras theorem,Example 6.4 hy poAopposite sidecosecA 24252, then find all the other trigonometric ratios.3AIf tan A Solution sin A hypotenuseopposite side of angle θsinq 13qA5Badjacent side of angle θFig. 6.8Standard Mathematics23-02-2019 14:18:56
132 52169 25 144 12AC 12AB 5 ; cos q BC 13BC 13Therefore, sinq 12524 152 32 sin q 3 cos q1313 13 9 3 RHSLHS48 45 34 sin q 9 cos q 4 12 9 5131313Note: We can also take the angle ‘ q ’ at the vertex ‘C’ and proceed in the same way.Exercise 6.11.From the given figure, find all the trigonometric ratios of angle B.40A9B41C2.3.4.5.6.7.AFrom the given figure, find the values of(i) sin B (ii) sec B(iii) cot B(iv) cosC (v) tanC(vi) cosecC13B5DC16If 2 cos q 3 , then find all the trigonometric ratios of angle q .3sin A cos A, then find the value of.52 tan A2xIf cos A, then find the values of sinA and tanA in terms of x.1 x2aIf sinq, then show that b sin q a cos q .22a b4 sin A 3 cos AIf 3 cot A 2 , then find the value of.2 sin A 3 cos AIf cos A C8 cos q 2 sin q.4 cos q 2 sin qq f8.If cos q : sin q 1 : 2, then find the value of9.From the given figure, prove that θ φ 90 .Also prove that there are two other right angledtriangles. Find sin a, cos b and tanf .152012aAb9D16BTrigonometry 2276 Trigonometry.indd 22723-02-2019 14:19:14
A boy standing at a point O finds his kite flying at a point P with distance OP 25m.10.It is at a height of 5m from the ground. When the thread is extended by 10m from P,it reaches a point Q. What will be the height QN of the kite from the ground? (usetrigonometric ratios)10mP25mQh5mOMN6.2 Trigonometric Ratios of Some Special AnglesThe values of trigonometric ratios of certain angles can be obtained geometrically.Two special triangles come to help here.C6.2.1 Trigonometric ratios of 45 45 Consider a triangle ABC with angles 45 , 45 and 90 asshown in the figure 6.9.It is the shape of half a square, cut along the square’sdiagonal. Note that it is also an isosceles triangle (both legs havethe same length, a units).a 2a45 ABaFig. 6.9Use Pythagoras theorem to check if the diagonal is oflength a 2 .Now, from the right-angled triangle ABC, sin 45oopposite side BCa hypotenuse AC a 2cos 45oadjacent side ABa hypotenuseAC a 2 tan45oopposite side BC a 1adjacent side AB a121The reciprocals of these ratiocan be easily foundout to becosec 45 2 ;2sec 45 2 andcot 45 1P6.2.2 Trigonometric Ratios of 30 and 60 Consider an equilateral triangle PQR of side length 2 units.30Draw a bisector of P. Let it meet QR at M.2Knowing PQ and QM, we can find PM, using Pythagorastheorem,6 Trigonometry.indd 22823PQ QR RP 2 units.QM MR 1 unit (Why?)228 60 Q1M1RFig. 6.109th Standard Mathematics23-02-2019 14:19:19
we find that PM 3 units.Now, from the right-angled triangle PQM,opposite side QM 1 hypotenusePQ 2adjacent side PM3cos 30o hypotenusePQ2opposite side QM1tan30o adjacent side PM3 sin30oThe reciprocals of these ratiocan be easily foundout to be2cosec 30 2, sec 30 3and cot 30 3We will use the same triangle but the other angle of measure 60 now.sin 60o cos 60o tan 60o opposite sidehypotenuseadjacent sidehypotenuseopposite sideadjacent side PMPQQMPQPMQM 3The reciprocals of these ratiocan be easily foundout to be212cosec602313and cot 60 313Y6.2.3 Trigonometric ratios of 0 and 90 To find the trigonometric ratios of0 and 90 , we take the help of what isknown as a unit circle.A unit circle is a circle of unit radius(that is of radius 1 unit), centred at theorigin.; sec 60 2P(cos q , sin q )sinqqOQcos qXWhy make a circle where the radiusis 1unit?This means that every referencetriangle that we create here has ahypotenuse of 1unit, which makes it somuch easier to compare angles and ratios.We will be interested only in thepositive values since we consider ‘lengths’and it is hence enough to concentrate onthe first quadrant.Fig. 6.11BP (x , y )1yqOQxFig. 6.12ATrigonometry 2296 Trigonometry.indd 22923-02-2019 14:19:26
We can see that if P(x,y) be any point on the unit circle in the first quadrant andPOQ qPQ yOQ xPQ y y ; cos q ; tan q x OP 1OP 1OQ xsin qWhen q 0 , OP coincides with OA, where A is (1,0) giving x 1, y 0 .We get thereby,sin 0 0 ;cos 0 1 ;tan 0 01 0 ;cosec 0 not defined (why?)sec 0 1cot 0 not defined (why?)When q 90 , OP coincides with OB, where B is (0,1) giving x 0, y 1 .Hence,sin90 1 ; cosec90 1cos 90 0 ;sec90 not defined1tan90 not defined ;cot 90 00Let us summarise all the results in the table given below:2306 Trigonometry.indd 230q0 30 45 60 sinq0121cos q123212tanq013cosecqnot definedsecq1cot qnot definedTrigonometricratio32132221232312321390 10notdefined1notdefined09th Standard Mathematics23-02-2019 14:19:43
Example 6.5(iii)Evaluate:(i) sin 30 cos 30Notetan 45tan 30 tan 602(ii) tan 60 cot 60 (i) (sin q )2 is written as sin2 q sin q sin q2(iv) sin 45 cos 45(ii) (sin q )2 is not written as sinq 2 , becauseit may mean as sin (q q ) .Solution31 31(i) sin 30 cos 3022 211(ii) tan 60 cot 60 33111tan 453 (iii)21 3tan 30 tan 604131 333 132212121 11 1 22(iv) sin 45 cos 451 222 22 2 22Thinking CornerThe set of three numbers are called as Pythagorean triplets as they form the sidesof a right angled triangle. For example,(i) 3, 4, 5(ii) 5, 12, 13(iii) 7, 24, 25Multiply each number in any of the above Pythagorean triplet by a non-zeroconstant. Verify whether each of the resultant set so obtained is also a Pythagoreantriplet or not.Example 6.6(i)Find the values of the following:(cos 0 sin 45 sin 30 )(sin 90 cos 45 cos 60 )3(ii) tan2 60 2 tan2 45 cot 2 30 2 sin2 30 cosec2 454Solution(i) (cos 0 sin 45 sin 30 )(sin 90 cos 45 cos 60 )1 1 1 1 1 1 2 2 2 2 6 Trigonometry.indd 231Trigonometry 23123-02-2019 14:19:56
2 2 2 2 2 2 2 2 3 2 2 3 2 2 2 22 2 2 2 2 2 18 414724 2 44 23(ii) tan2 60 2 tan2 45 cot 2 30 2 sin2 30 cosec2 4542222 1 323 2(1)2 3 2 2 41 33 2 32 242 2 2 02Note(i) In a right angled triangle, if the angles are in the ratio 45 : 45 : 90 , then thesides are in the ratio 1 : 1 : 2 .(ii) Similarly, if the angles are in the ratio 30 : 60 : 90 , then the sides are in theratio 1 : 3 : 2 .(The two set squares in your geometry box is one of the best example for the above twotypes of triangles).Exercise 6.21.2.Verify the following equalities:(i) sin2 60 cos2 60 1(iii)cos 90 1 2 sin2 45 2 cos2 45 1(ii) 1 tan2 30 sec2 30(iv)sin 30 cos 60 cos 30 sin 60 sin 90Find the value of the following:(i)tan 45 sec 60 5 sin 90cosec30 cot 45 2 cos 0(ii) (sin 90 cos 60 cos 45 ) (sin 30 cos 0 cos 45 )(iii) sin2 30 2 cos3 60 3 tan 4 453.Verify cos 3 A 4 cos3 A 3 cos A , when A 304.Find the value of 8 sin 2 x cos 4 x sin 6 x, when x 152326 Trigonometry.indd 2329th Standard Mathematics23-02-2019 14:20:05
6.3 Trigonometric Ratios for Complementary AnglesCqRecall that two acute angles are said to be complementary ifthe sum of their measures is equal to 90 .What can we say about the acute angles of a right-angledtriangle?In a right angled triangle the sum of the two acute angles is Aequal to 90 . So, the two acute angles of a right angled triangle arealways complementary to each other.90 qFig. 6.13BIn the above figure 6.13, the triangle is right-angled at B. Therefore, if C is q , then A 90 q .We find thatABACBCcos qACABtan qBCsin qSimilarly for the angle ( 90 – θ ), We haveACABACsec q.(1)BCBCcot qAB cosec qBCACABcos(90 q )ACBCtan(90 q )ABsin(90 q )ACBC AC sec(90 q ) .(2)AB AB cot(90 q )BC cosec(90 q )Comparing (1) and (2), we getsinq cos(90 q )cosecq sec(90 q )cos q sin(90 q )secq cosec(90 q )tanq cot(90 q )cot q tan(90 q )Example 6.7Express (i) sin74 in terms of cosine (ii) tan12 in terms ofcotangent (iii) cosec39 in terms of secantSolution(i)sin74 sin(90 16 )(since, 90 16 74 )RHS is of the form sin(90 q ) cos qTherefore sin74 cos16(ii) tan12 tan(90 78 )(since, 12 90 78 )RHS is of the form tan(90 q ) cot qTherefore tan12 cot 78Trigonometry 2336 Trigonometry.indd 23323-02-2019 14:20:18
(iii) cosec39 cosec(90 51 )(since 39 90 51 )RHS is of the form cosec(90 q ) sec qTherefore cosec39 sec51Example 6.8Evaluate:(i)Solution(i)sin 49 cos 41 (ii)sec63 cosec 27 sin 49 cos 41 sin 49 sin(90 41 ) cos 41 , since 49 41 90 (complementary),cos 41Hence on substituting sin 49 cos 41 we get,1cos 41sec63 (ii)cosec 27 sec 63 sec(90 27 ) cosec 27 , here 63 and 27 are complementary angles.we haveExample 6.9cosec 27sec631cosec 27 cosec 27Find the values of (i) tan 7 tan 23 tan 60 tan 67 tan 83 (ii)Solution(i)cos 35 sin 12 cos 18sin 55 cos 78 sin 72tan 7 tan 23 tan 60 tan 67 tan 83 tan 7 tan 83 tan 23 tan 67 tan 60(Grouping complementary angles)tan 7 tan(90 7 )tan 23 tan(90 23 )tan 60(tan 7 .cot 7 )(tan 23 .cot 23 )tan 60(1) (1) tan 60tan60(ii)2346 Trigonometry.indd 234 3cos 35 sin 12 cos 18sin 55 cos 78 sin 72 since cos(90 55 ) sin(90 78 ) cos(90 72 ) cos 35 cos(90 55 ) sin 12 sin(90 78 ) sin 55cos 78sin 72 cos 18 cos(90 72 ) 9th Standard Mathematics23-02-2019 14:20:27
sin 55 cos 78 sin 72sin 55 cos 78 sin 721 1 1 1Example 6.10(i) If cosec A sec 34 , then find A (ii) If tan B cot 47 , then find B.SolutionWe know that cosec A sec(90 A)(i)(ii) We know that tan B cot(90 B)cot(90 B) cot 47sec(90 A) sec(34 )90 B 4790 A 34B 90 47 We getA 90 34 We getB 43A 56Exercise 6.3Find the value of the following:22cos 70 cos 59cos qcos 47 sin 72 (i) 2 cos2 45 (ii)8 cos2 60 sin 20 sin 31 sin(90 q )sin 43 cos 18 (iii) tan 15 tan 30 tan 45 tan 60 tan 75 (iv)cot qcos(90 q )tan q sec(90 q ) tan(90 q ) sin(90 q )cot(90 q )cosec(90 q )Thinking Corner(i) What are the minimum and maximum values of sinq ?(ii) What are the minimum and maximum values of cos q ?6.4 Method of using Trigonometric TableWe have learnt to calculate the trigonometric ratios for angles 0 , 30 , 45 , 60 and90 . But during certain situations we need to calculate the trigonometric ratios of all theother acute angles. Hence we need to know the method of using trigonometric tables.One degree (1 ) is divided into 60 minutes ( 60′ ) and one minute ( 1′ ) is divided into60 seconds ( 60′′ ). Thus, 1 60′ and 1 60 .Trigonometry 2356 Trigonometry.indd 23523-02-2019 14:20:35
The trigonometric tables give the values, correct to four places of decimals for theangles from 0 to 90 spaced at intervals of 60′ . A trigonometric table consists of three parts.A column on the extreme left which contains degrees from 0 to 90 , followed by tencolumns headed by 0′ , 6′ , 12′ , 18′ , 24′ , 30′ , 36′ , 42′ , 48′ and 54′ .Five columns under the head mean difference has values from 1,2,3,4 and 5.For angles containing other measures of minutes (that is other than 0′ , 6′ , 12′ , 18′ ,24′ , 30′ , 36′ , 42′ , 48′ and 54′ ), the appropriate adjustment is obtained from the meandifference columns.The mean difference is to be added in the case of sine and tangent while it is to besubtracted in the case of cosine.Now let us understand the calculation of values of trigonometric angle from thefollowing examples.Example 6.11Find the value of sin 64 34 .Solution0′6′ 12′ 18′ 24′0.0 0.1 0.2 0.3 0.4 64 30′0.5 36′ 42′ 48′ 54′0.6 0.7 0.8 0.9 0.9026Mean Difference123455Write 64 34 64 30 4From the table we have, sin64 30 0.9026Mean difference forExample 6.124′ 5(Mean difference to be added for sine)sin64 34 0.9031Find the value of cos19 59Solution0′6′ 12′ 18′ 24′ 30′ 36′ 42′ 48′0.0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 19 54′0.9 0.9403Mean Difference1 2 3 4 55Write 19 59 19 54 5From the table we have,cos19 54 0.9403Mean difference for2366 Trigonometry.indd 2365′ 5 (Mean difference to be subtracted for cosine)cos19 59 0.93989th Standard Mathematics23-02-2019 14:20:47
Example 6.13Find the value of tan70 13Solution0′6′0.0 0.1 70 24′30′36′42′48′54′Mean Difference12′18′0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 12.7776234526Write 70 13 70 12 1From the table we have, tan70 12 2.77761′ Mean difference for26 (Mean difference to be added for tan)tan70 13 2.7802Example 6.14Find the value of(i) sin 38 36 tan 12 12(ii) tan 60 25 cos 49 20Solution(i)sin 38 36 tan 12 12(ii)tan 60 25 cos 49 20sin38 36 0.6239tan60 25 1.7603 0.0012 1.7615tan12 12 0.2162cos 49 20 0.6521 0.0004 0.6517tan 60 25 cos 49 20 1.1098sin38 36 tan12 12 0.8401Example 6.15Find the value of q if(ii) cos q 0.7656(i) sin q 0.9858Solution(i) sin q 0.9858 0.9857 0.0001From the sine table 0.9857 80 18Mean difference 1 2′0.9858 80 20sin q 0.9858 sin80 20q 80 20(ii) cos q 0.7656 0.7660 0.0004From the cosine table0.7660 40 0′Mean difference 4 2′0.7656 40 2′cos q 0.7656 cos 40 2′q 40 2′Trigonometry 2376 Trigonometry.indd 23723-02-2019 14:20:59
Example 6.16Find the area of the right angled triangle with hypotenuse 5cm andone of the acute angle is 48 30SolutionFrom the figure,ABACABsin 48 30 5AB0.7490 55 0.7490 ABBCACBCcos 48 30 5BC0.6626 50.6626 5 BCsinq Acos q 5cm48 30BBC 3.313 cmAB 3.7450 cmFig. 6.14C1Area of right triangle bh21BC AB213.3130 3.745021.6565 3.7450 6.2035925 cm2ActivityObserve the steps in your home. Measure the breadth and the height of one step.Enter it in the following picture and measure the angle (of elevation) of that step.AAhhB(i)(ii)2386 Trigonometry.indd 238bCBbqCCompare the angles (of elevation) of different steps of same height and samebreadth and discuss your observation.Sometimes few steps may not be of same height. Compare the angles (ofelevation) of different steps of those different heights and same breadth anddicuss your observation.9th Standard Mathematics23-02-2019 14:21:05
Exercise 6.41.Find the value of the following:(i) sin 49 (ii) cos 74 39 (iii) tan54 26 (iv) sin21 21 (v) cos 33 53 (vi) tan70 172.3.Find the value of q if(i) sin q 0.9975(ii) cos q 0.6763(iv) cos q 0.0410(v) tan q 7.5958(iii) tan q 0.0720Find the value of the following:(i) sin 65 39 cos 24 57 tan 10 10(ii) tan 70 58 cos 15 26 sin 84 594.Find the area of a right triangle whose hypotenuse is 10cm and one of the acuteangle is 24 245.Find the angle made by a ladder of length 5mwith the ground, if one of its end is 4m awayfrom the wall and the other end is on the wall.6.HIn the given figure, HT shows the height of atree standing vertically. From a point P, theangle of elevation of the top of the tree (that is P ) measures 42 and the distance to the treeis 60 metres. Find the height of the tree.42 T60 mPExercise 6.5Multiple choice questions1.2.3.4.If sin30 x and cos 60 y , then x 2 y 2 is1(1)(2) 0(3) sin90 2(4) cos 90 If tan q cot 37 , then the value of q is(1) 37 (2) 53 (3) 90 (4) 1 The value of tan 72 tan18 is(1) 0(2) 1(4) 72 The value of(1) cos 60 (3) 18 2 tan 30is equal to1 tan2 30(2) sin60 (3) tan60 (4) sin30 Trigonometry 2396 Trigonometry.indd 23923-02-2019 14:21:10
If 2 sin 2q 3 , then the value of q is5.(1) 90 (2) 30 (3) 45 (4) 60 The value of 3 sin 70 sec 20 2 sin 49 sec 51 is6.(1) 27.(2) 3(3) 5(4) 61 tan2 45The value ofis1 tan2 45(1) 2(2) 1(3) 0(4)(1) 0(3) 2(4) 312The value of cosec(70 q ) sec(20 q ) tan(65 q ) cot(25 q ) is8.9.(2) 1The value of tan1 tan 2 tan 3 . tan 89 is(1) 0(2) 1(3) 2(4)3211and cos b , then the value of α β is22(2) 90 (3) 30 (4) 60 Given that sina 10.(1) 0 Points to Rememberzz Trigonometric ratios areopposite sidesinθ hypotenuseadjacent sidecos θ tan θ hypotenuseopposite sideadjacent sidecosec θ hypotenuseopposite sidesec θ hypotenuseadjacent sidecot θ adjacent sideopposite sidezz Reciprocal trigonometric ratios1cos ec q1cosec θ sinqzz Complementary anglessinθ 2406 Trigonometry.indd 2401sec q1sec θ cos q1cot q1cot θ tanqcos θ tan θ sinq cos(90 q )cosecq sec(90 q )cos q sin(90 q )secq cosec(90 q )tanq cot(90 q )cot q tan(90 q )9th Standard Mathematics23-02-2019 14:21:13
ICT CornerExpected Result is shownin this pictureStep – 1Open the Browser by typing the URL Link given below (or) Scan the QR Code. GeoGebra worksheet named “Trigonometry” will open. There are three worksheets under the title Trigonometricratios and Complementary angles and kite problem.Step - 2Move the sliders of the respective values to change the points and ratio. Work out the solution andcheck.For the kite problem click on “NEW PROBLEM” to change the question and work it out. Click thecheck box for solution to check your answer.Step 1Step 2Scan the QR Code.Trigonometry 2416 Trigonometry.indd 24123-02-2019 14:21:13
2426 Trigonometry.indd 242DegreeNATURAL SINES0 6 12 18 24 30 36 42 48 54 Mean Difference0.0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 .69340.70592222244444776669998811111111109th Standard Mathematics23-02-2019 14:21:14
NATURAL SINES6 12 18 24 30 36 42 48 54 Mean DifferenceDegree0 0.0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 000000000110001110011100Trigonometry 2436 Trigonometry.indd 24323-02-2019 14:21:15
NATURAL COSINES(Numbers in mean difference columns to be subtracted, not added)2446 Trigonometry.indd 2446 12 18 24 30 36 42 48 54 Mean DifferenceDegree0 0.0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 98950.98690.99560.99380.99170.98930.98
adjacent side of angle θ e q adjacent side of angle θ q Relationship sinθ oppositeside hypotenuse cosθ adjacent side hypotenuse tanθ oppositeside adjacent side Fig. 6.2 x units 10.5 units 35 C B A A A C C C B B opposite side B of angle θ opposite side of angle θ A C